This is incredible. In 2005, mathematician Mike Keith took a 717-word section from the essay on Mount Fuji in Lafcadio Hearn’s 1898 Exotics and Retrospective and anagrammed it into nine 81-word poems, each inspired by an image from Hokusai’s famous series of landscape woodcuts, the Views of Mount Fuji.
That’s not the most impressive part. Each anagrammed poem can be arranged into a 9 × 9 square, with one word in each cell. Stacking the nine grids produces a 9 × 9 × 9 cube. Make two of these cubes, and then:
- In Cube “D” (for Divisibility), assign each cell the number “1” if the sum of the letter values in the corresponding word (using A=1, B=2, C=3 etc.) is exactly divisible by 9, or “0” if it is not.
- In Cube “L” (for Length), assign each cell the number “1” if its word has exactly nine letters, or “0” if it does not.
Replace each “1” cell with solid wood and each “0” cell with transparent glass. Now suspend the two cubes in a room and shine beams of light from the top and right onto Cube D and from the front and right onto Cube L:
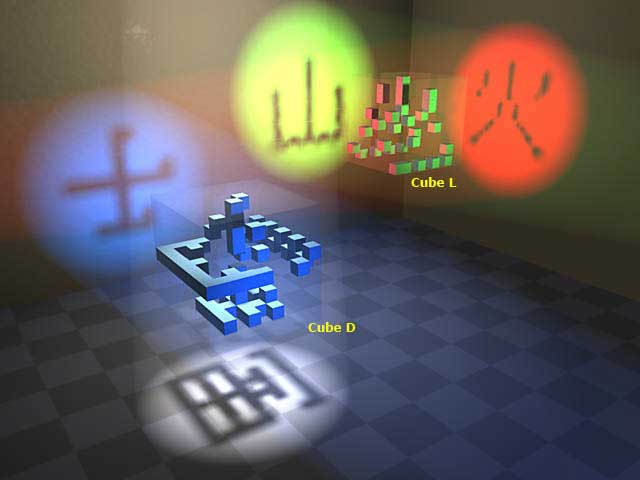
The shadows they cast form reasonable renderings of four Japanese kanji characters relevant to the anagram:
The red shadow is the symbol for fire.
The green shadow is the symbol for mountain.
Put together, these make the compound Kanji symbol (“fire-mountain”) for volcano.
The white shadow is the symbol for wealth, pronounced FU
The blue shadow is the symbol for samurai, pronounced JI
Put together, these make the compound word Fuji, the name of the mountain.
See Keith’s other anagrams, including a 211,000-word recasting of Moby-Dick.