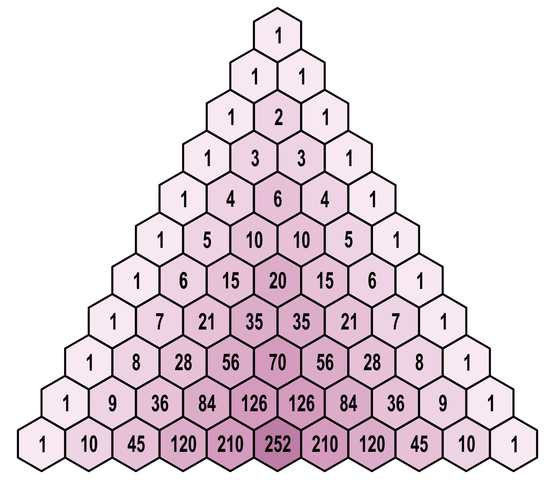
The product of the six numbers surrounding any interior number in Pascal’s triangle is a perfect square.
A Square Triangle
Science & Math
Misc
- ZZ Top’s first album is called ZZ Top’s First Album.
- Supreme Court justice Byron White was the NFL’s top rusher in 1940.
- LOVE ME TENDER is an anagram of DENVER OMELET.
- Every palindromic number with an even number of digits is divisible by 11.
- “In great attempts it is glorious even to fail.” — Cassius
From English antiquary John Aubrey’s 1696 Miscellanies: “Anno 1670, not far from Cyrencester, was an Apparition; Being demanded, whether a good Spirit or a bad? Returned no answer, but departed with a curious Perfume and a most melodious Twang.”
Mirror Multiplication
203313 × 657624 = 426756 × 313302
Love and Laureates
George Hitchings, who won the Nobel Prize in medicine in 1988, proposed to his wife by saying “Incidentally, you’re my fiancée now” as they drove to an event.
John Bardeen, who won the prize in physics in both 1956 and 1972, told his fiancée, “You can be married in the church if you want to, but not to me.”
Hemingway, a Nobelist in literature in 1954, said, “I remember after I got that marriage license I went across from the license bureau to a bar for a drink. The bartender said, ‘What will you have, sir?’ And I said, ‘A glass of hemlock.'”
And Wolfgang Pauli won the Nobel in physics in 1945. Of his ex-wife’s remarriage, he said, “Had she taken a bullfighter I would have understood, but an ordinary chemist!”
The Tipping Point
English meteorologist Lewis Fry Richardson (1881-1953) spent the last 25 years of his life trying to establish a mathematical theory of the causes of war. In the first of two books on this subject, Arms and Insecurity, he works out a model of arms races using differential equations and reaches the conclusion that
![\frac{d\left ( U + V \right )}{dt}=\left ( k - \alpha \right )\left \{ U + V - \left [ U_{0} + V_{0} - \frac{g + h}{k - \alpha } \right ] \right \}](https://s0.wp.com/latex.php?latex=+%5Cfrac%7Bd%5Cleft+%28+U+%2B+V+%5Cright+%29%7D%7Bdt%7D%3D%5Cleft+%28+k+-+%5Calpha++%5Cright+%29%5Cleft+%5C%7B+U+%2B+V+-+%5Cleft+%5B+U_%7B0%7D+%2B+V_%7B0%7D+-+%5Cfrac%7Bg+%2B+h%7D%7Bk+-+%5Calpha+%7D+%5Cright+%5D+%5Cright+%5C%7D+&bg=ffffff&fg=000&s=2&c=20201002)
where:
U and V are the annual defense budgets of two parties to a conflict
k is a positive constant representing the response to threat
α is a positive constant representing the fatigue and expense of keeping up defenses
U0 and V0 represent cooperations between the parties, tentatively assumed to remain constant
and g and h represent the “grievances and ambitions, provisionally regarded as constant,” on each side.
The term in brackets is a constant, so Richardson predicted that plotting d(U + V)/dt against (U + V) would produce a straight line. He tried this out using the defense budgets of the Franco-Russian and Austro-German alliances for 1909-14 and got this:
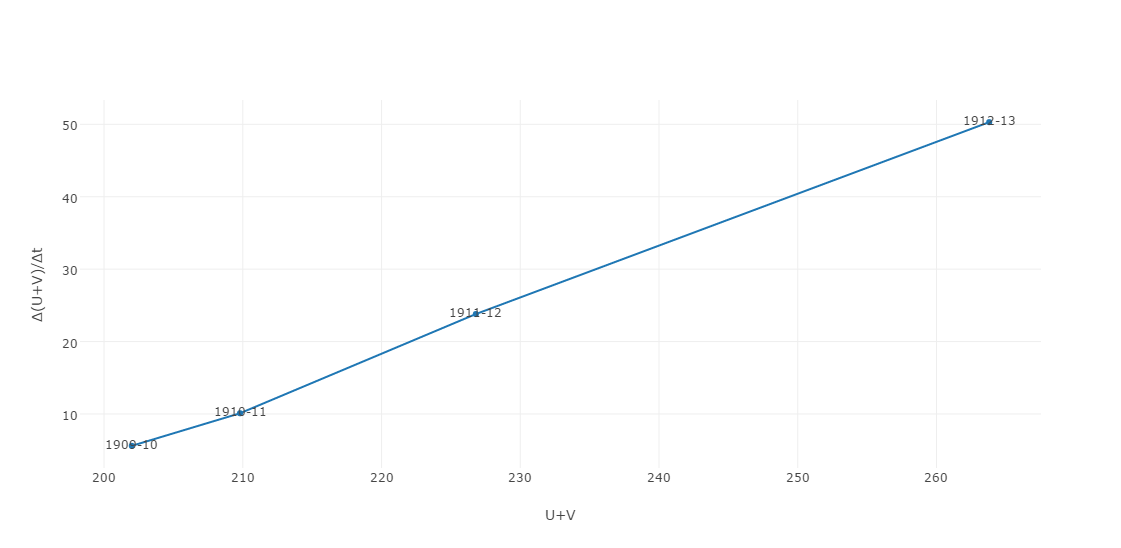
“The four points lie close to a straight line, closer, indeed, than one might expect,” he writes. “Since I first drew this diagram, which was shown at the British Association in Cambridge in 1938, and printed in Nature of 29 October of that year, I have been incredulous about the marvelously good fit. Yet there is no simple mistake. … The mere regularity of these phenomena shows that foreign politics had then a rather machine-like quality, intermediate between the predictability of the moon and the freedom of an unmarried young man.”
The extrapolated straight line hits the x axis at U + V = £194 pounds sterling. “As love covereth a multitude of sins, so the good will between the opposing alliances would just have covered £194 million of defense expenditures on the part of the four nations concerned. Their actual expenditure in 1909 was £199 millions; and so began an arms race which led to World War I.”
(Lewis F. Richardson, Arms and Insecurity, 1949.)
Unquote
“In questions of science, the authority of a thousand is not worth the humble reasoning of a single individual.” — Galileo
Blackwell’s Bet
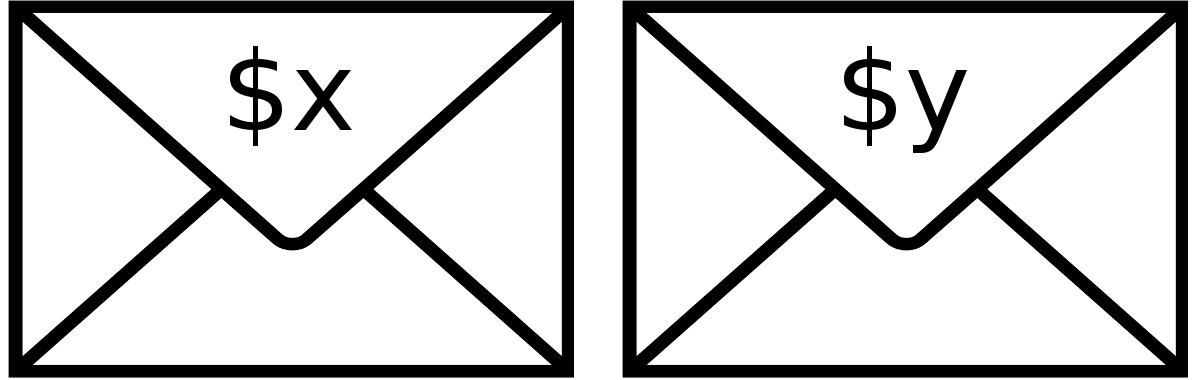
Two envelopes contain unequal sums of money (for simplicity, assume the two amounts are positive integers). The probability distributions are unknown. You choose an envelope at random, open it, and see that it contains x dollars. Now you must predict whether the total in the other envelope is more or less than x.
Since we know nothing about the other envelope, it would seem we have a 50 percent chance of guessing correctly. But, El Camino College mathematician Leonard Wapner writes, “Unexpectedly, there is something you can do, short of opening the other envelope, to give yourself a better than even chance of getting it right.”
Choose a random positive integer, d, by any means at all. (If d = x then choose again until this isn’t the case.) Now if d > x, guess more, and if d < x, guess less. You’ll guess correctly more than 50 percent of the time.
How is this possible? The random number is chosen independently of the envelopes. How can it point in the direction of the unknown y most of the time? “Think of it this way,” writes Wapner. “If d falls between x and y then your prediction (as indicated by d) is guaranteed to be correct. Assume this occurs with probability p. If d falls less than both x and y, then your prediction will be correct only in the event your chosen number x is the larger of the two. There is a 50 percent chance of this. Similarly, if d is greater than both numbers, your prediction will be correct only if your chosen number is the smaller of the two. This occurs with a 50 percent probability as well.”
So, on balance, your overall probability of being correct is
That’s greater than 0.5, so the odds are in favor of your making a correct prediction.
This example is based on a principle identified by Stanford statistician David Blackwell. “It’s unexpected and ironic that an unrelated random variable can be used to predict that which appears to be completely unpredictable.”
(Leonard M. Wapner, Unexpected Expectations: The Curiosities of a Mathematical Crystal Ball, 2012, following David Blackwell, “On the Translation Parameter Problem for Discrete Variables,” Annals of Mathematical Statistics 22:3 [1951], 393–399.)
Presto
Raymond Smullyan offered this proof at a conference on self-reference:
A: Santa Claus exists, if I am not mistaken.
B: Well, of course Santa Claus exists if you are not mistaken!
A: So I was right.
B: Yes.
A: So I was not mistaken.
B: Yes.
A: Hence, Santa Claus exists.
Paean
Proctologist W.C. Bornemeier explains why the sphincter ani must be preserved when performing hemorrhoid surgery:
They say man has succeeded where the animals fail because of the clever use of his hands, yet when compared to the hands, the sphincter ani is far superior. If you place into your cupped hands a mixture of fluid, solid and gas and then through an opening at the bottom, try to let only the gas escape, you will fail. Yet the sphincter ani can do it. The sphincter apparently can differentiate between solid, fluid and gas. It apparently can tell whether its owner is alone or with someone, whether standing up or sitting down, whether its owner has his pants on or off. No other muscle in the body is such a protector of the dignity of man, yet so ready to come to his relief. A muscle like this is worth protecting.
— W.C. Bornemeier, “Sphincter Protecting Hemorrhoidectomy,” American Journal of Proctology 11 (1960), 48-52
The Return of Monty Hall
In 2003, Danish computer scientist Peter Bro Miltersen discussed a surprisingly effective technique by which a player might guess the colors of slips of paper hidden in boxes (PDF). As this circulated in the mathematical community it evolved into a puzzle in which a group of 100 prisoners must find their own names on slips of paper. I wrote about it in 2011.
When Eugene Curtin and Max Warshauer wrote about the prisoner puzzle in The Mathematical Intelligencer in December 2006, reader A.S. Landsberg offered a variant called “The Return of Monty Hall.” On a new game show for couples, there are three curtains, which hide a key, a car, and a goat. One member of the couple is the “car-master” — she must find the car. The other is the “key-master” — he must find the key. If both succeed in their tasks, they win the new car. If either fails, they win the goat.
The key-master is led out of the room, where he can’t observe the proceedings, and then the car-master has two tries to find the car (open one curtain, and if the car isn’t there, open another curtain). If she finds the car, then all the curtains are closed again and the key-master is brought on to find the key. No communication at all is permitted between the two at this point. As before, the key-master has two tries to find the key by opening curtains.
If the couple play optimally, their odds of winning the car are a surprising 2/3. They do this using Miltersen’s technique. The car-master is Player #1, the key-master is Player #2, the car is Prize #1, the key is Prize #2, and the goat is Prize #3. The strategy is simply for each player to start by opening the curtain corresponding to his or her own player number, and if unsuccessful to open the curtain number corresponding to the prize number that the first curtain reveals. So, for example, the car-master, who is Player #1, begins by opening Curtain #1. If she finds the car then she’s done; if she finds the key (Prize #2) then she opens Curtain #2, and if she finds the goat (Prize #3) then she opens Curtain #3. When the curtains are reclosed, the key-master begins his turn by opening Curtain #2 (since he’s Player #2) and following the same plan.
That’s it. It’s not guaranteed to work, but it’s a simple strategy that requires minimal preparation and no communication at all once the game has begun. The universe of possibilities is so small that we can simply count them — here are the various arrangements of prizes and the resulting outcomes:
car-key-goat: win
car-goat-key: win
key-goat-car: lose
key-car-goat: win
goat-key-car: win
goat-car-key: lose
Landsberg’s letter brought a comment by reader Eric Grunwald, who pointed out that a third person can be introduced to the Monty Hall game without reducing the overall chance of success. Replace the goat with a GPS system and add a third contestant, the “GPS-master.” Following the same rules, and again forbidding any communication among the contestants, Miltersen’s strategy ensures a 2/3 probability that all three players find their prizes.