Science & Math
A Better Nature
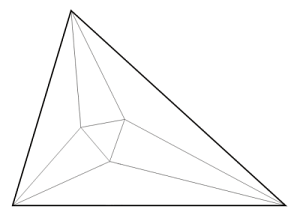
Trisect the angles of any triangle and you’ll find an equilateral triangle at its heart.
This theorem has a curious cousin: If you trisect the sides of any triangle and erect an equilateral triangle outwardly on the middle third of each leg, then the outermost vertices of these equilateral triangles will themselves form an equilateral triangle.
The Thatcher Effect
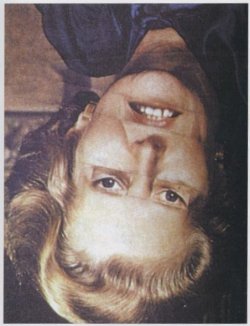
When we look at another person’s face, her eyes and mouth convey the most information about her mood.
Indeed, when a face is inverted we can have trouble recognizing it because we can’t read its expression.
So in 1980 University of York psychologist Peter Thompson tried inverting everything but the eyes and mouth.
Most people can recognize the face at left and assign a mood to it, but they’re often surprised to see it right side up.
“Further research into this illusion might help determine whether face recognition is a serial or parallel process,” Thompson wrote in Perception that summer. “It might even tell us something about Margaret Thatcher.”
Scholars and Sense
O Cleinias, are those who learn the wise or the ignorant?
He answered that those who learned were the wise.
Euthydemus proceeded: There are some whom you would call teachers, are there not?
The boy assented.
And they are the teachers of those who learn — the grammar-master and the lyre master used to teach you and other boys; and you were the learners?
Yes.
And when you were learners you did not as yet know the things which you were learning?
No, he said.
And were you wise then?
No, indeed, he said.
But if you were not wise you were unlearned?
Certainly.
You then, learning what you did not know, were unlearned when you were learning?
The youth nodded assent.
Then the unlearned learn, and not the wise, Cleinias, as you imagine.
— Plato, Euthydemus
Math Notes
Never Mind
“It has been asserted (by C.S. Lewis, for instance) that no determinist rationally can believe in determinism, for if determinism is true, his beliefs were caused, including his belief in determinism. The idea seems to be that the causes of belief, perhaps chemical happenings in the brain, might be unconnected with any reasons for thinking determinism true. They might be, but they need not be. The causes might ‘go through’ reasons and be effective only to the extent that they are good reasons.”
— Robert Nozick, “Reflections on Newcomb’s Paradox,” 1974
“If … [determinism] is true, then the intellectual or cognitive operations of its upholders, including their choice or decision to maintain the thesis, … are themselves only the effects of inexorable forces. But if this is so, why should the thesis … be accepted as valid or true?”
— Alan Gewirth, Reason and Morality, 1978
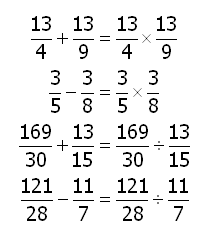
Pandigital Approximations
The digits 1-9 can work some impressive tricks:

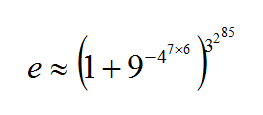
The first formula, found by B. Ziv in 2004, produces the first 10 digits of pi.
The second, astonishingly, reproduces e to 18,457,734,525,360,901,453,873,570 decimal places. It was discovered by Richard Sabey, also in 2004.
(Thanks, Robin.)
Party of One

“A man wrote to say that he accepted nothing but Solipsism, and added that he had often wondered it was not a more common philosophy. Now Solipsism simply means that a man believes in his own existence, but not in anybody or anything else. And it never struck this simple sophist, that if his philosophy was true, there obviously were no other philosophers to profess it.”
— G.K. Chesterton, St. Thomas Aquinas, 1933
Digit Acrobatics
214358976 = (3 + 6)2 + (4 + 7)8 + (5 + 9)1
“Triangle Rhyme”
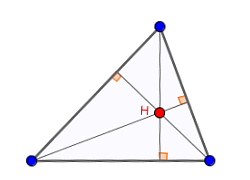
Although the altitudes are three,
Remarks my daughter Rachel,
One point’ll lie on all of them:
The orthocenter H’ll.
By mathematician Dwight Paine of Messiah College, 1983.
(Further recalcitrant rhymes: month, orange. W.S. Gilbert weighs in.)
