Twice a year, objects Hawaii lose their shadows as the sun passes directly overhead.
A “zero shadow day” occurs biannually between the Tropics of Cancer and Capricorn, arriving at each location when the sun’s declination equals its latitude.
Twice a year, objects Hawaii lose their shadows as the sun passes directly overhead.
A “zero shadow day” occurs biannually between the Tropics of Cancer and Capricorn, arriving at each location when the sun’s declination equals its latitude.
What’s the funniest number? Yale physicist Emily Pottebaum proposed the Perceived Specificity Hypothesis, which states that “for nonnegative integers < 100, the funniness of a number increases with its apparent precision." She surveyed 68 acquaintances and found that:
The degree of specificity characterizes the distance between an integer and the nearest multiple of 5:
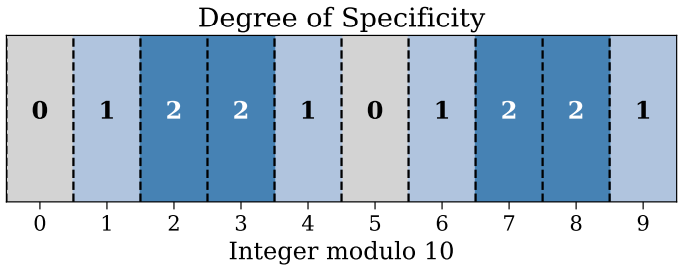
So 3, 7, 13, 17, etc. were judged to be funniest.
“I acknowledge my Ph.D. advisor, who I shall not name out of respect for her academic integrity, for her exasperation upon learning about this study. I thank her for putting up with my antics and plead that she continue to do so until I graduate.”
(E.G. Pottebaum, “What Is the Funniest Number? An Investigation of Numerical Humor,” arXiv preprint, arXiv:2503.24175 [2025].)
In the Spring 1957 issue of Pi Mu Epsilon Journal, C.W. Trigg points out that, by two continuous cuts, the surface of a cube can be divided into two pieces that can be unfolded and assembled into a hollow square:
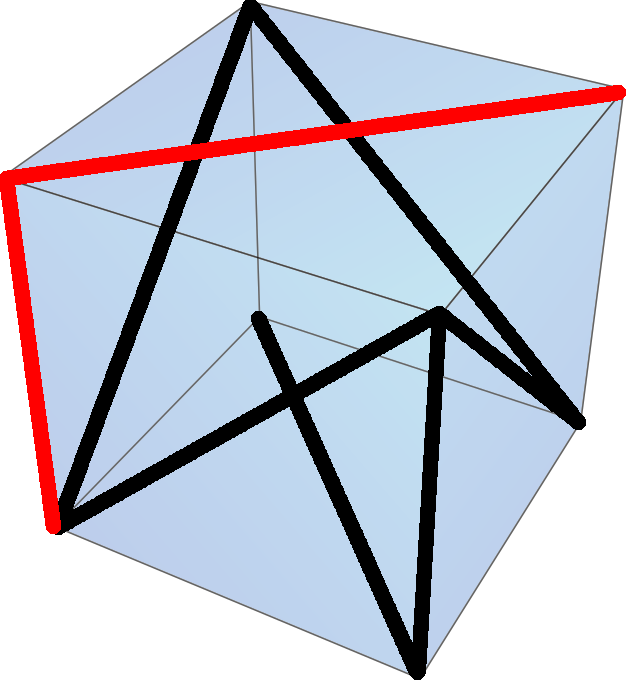
The cuts divide the cube’s surface into two congruent pieces, each composed of six connected isosceles right triangles. Joining these two pieces forms a hollow square with exterior side and interior side
, where x is the length of the cube’s edge.
10/10/2025 UPDATE: Reader Nick Hare made a much, much, much, much better diagram:
(Thanks, Nick.)
Two numbers are said to be betrothed if the sum of the proper divisors of each number is 1 more than the value of the other. For example:
The proper divisors of 48 are 1, 2, 3, 4, 6, 8, 12, 16, and 24. 1 + 2 + 3 + 4 + 6 + 8 + 12 + 16 + 24 = 76 = 75 + 1.
The proper divisors of 75 are 1, 3, 5, 15, and 25. 1 + 3 + 5 + 15 + 25 = 49 = 48 + 1.
Interestingly, in all such pairs discovered so far, one number is odd and the other even. Is this always the case? That’s an open question.
From Lee Sallows:
Numerical panmagic squares of 3×3 being impossible, the above square is in fact the first known order-3 panmagic square, a boast it can enjoy until the day that someone comes up with an improved solution. Such as one using all nine connected pieces, say.
Or not, perhaps? For the square above has a further property that other panmagic squares may not possess. Choose any three of the four corner pieces. There are four possibilities: aci, cig, agi and acg. Whatever your choice, the three pieces selected will tile the target.
(Thanks, Lee!)
A volunteer deals cards from an ordinary deck into two piles, stopping whenever he likes. He tosses aside one of the piles and peeks at the top card of the other pile. Then he drops the remainder of the cards on top of this pile and deals everything again into two piles. The magician, who has never touched the cards, now divines which of the two piles contains the memorized card and indeed discovers the card itself.
This trick is based on the Penelope principle, a mathematical idea worked out by Scottish magician and computer programmer Alex Elmsley. Elmsley’s technique required a perfect faro shuffle, so David Rutter revised it into a simpler version that’s entirely self-working. It was presented at the 15th Gathering for Gardner conference last year.
(Thanks, Joe.)
In 1831, in the appendix to a book on the raising of trees for shipbuilding, Scottish grain merchant Patrick Matthew suggested that, over great expanses of time, “the progeny of the same parents, under great difference of circumstance, might, in several generations, even become distinct species, incapable of co-reproduction.” He’d struck on the central idea of natural selection nearly 30 years before On the Origin of Species:
As the field of existence is limited and pre-occupied, it is only the hardier, more robust, better suited to circumstance individuals, who are able to struggle forward to maturity, these inhabiting only the situations to which they have superior adaptation and greater power of occupancy than any other kind; the weaker, less circumstance-suited, being prematurely destroyed.
On learning of this, Darwin allowed that Matthew “briefly but completely anticipates the theory of Nat. Selection.” In a letter to the Gardeners’ Chronicle, in which Matthew had pointed out his early observation, Darwin wrote, “I freely acknowledge that Mr. Matthew has anticipated by many years the explanation which I have offered of the origin of species, under the name of natural selection.”
But modern observers point out that Matthew didn’t pursue the idea and may not have realized the breadth of its import. Historian of science Peter Bowler writes, “Simple priority is not enough to earn a thinker a place in the history of science: one has to develop the idea and convince others of its value to make a real contribution.” Darwin called Matthew’s note “a complete but not developed anticipation … Anyhow one may be excused in not having discovered the fact in a work on Naval Timber.”
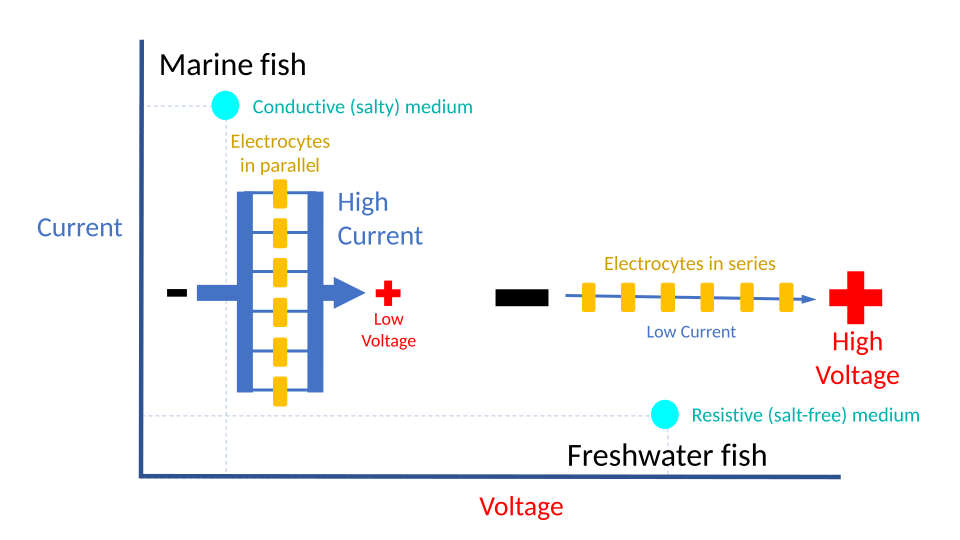
Among electric fish, those that live in freshwater have their generating cells arranged “in series”; in saltwater they appear “in parallel.”
Seawater conducts better than fresh water, so freshwater fish need to generate a high voltage to overcome the resistance of their medium. Their electricity-producing cells are arranged in series.
Marine fish operate at higher currents but lower voltages, so their electrocytes appear in parallel.