From reader Éric Angelini:
What is the smallest number that doesn’t have a 1 and yet has one?
From reader Éric Angelini:
What is the smallest number that doesn’t have a 1 and yet has one?
From reader Ian Duff:
“It is easy to establish that the self-descriptive phrase ‘this phrase contains thirty-five letters’ is the only such one with a correct count. No equivalent is possible in French or German, but in Italian questa frase contiene XX lettere, where XX is a number in word form, again has only one solution.”
What is it?

A problem by Russian mathematician Viktor Prasolov: This hexagon has been divided into black and white triangles so that any two triangles either have a common side (in which case they’re painted different colors) or a common vertex or they have no points in common, and each side of the hexagon is a side of one of the black triangles. Prove that no such partition exists for a 10-gon.
Alice and Bob are playing a game. An n×n checkerboard lies between them. Alice begins by marking a corner square, and thereafter the two of them take turns marking squares; each one they choose must be adjacent orthogonally to the last one chosen, so together they’re making a path around the board. When the path can’t continue (because no unmarked adjacent square is available), then the player who moved last wins. For which n can Alice devise a winning strategy? What if she has to start by marking a square adjacent to a corner, rather than the corner itself?
Visiting England’s Wirral Peninsula in 1853, Nathaniel Hawthorne came upon a queer battlemented house in the town of Bebington, “quite a novel symbol of decay and neglect,” “probably the whim of some half-crazy person.” “On the wall, close to the street, there were certain eccentric inscriptions cut into slabs of stone, but I could make no sense of them.”
The crazy person was resident Thomas Francis, and the inscriptions had apparently been commissioned to bemuse and entertain passersby. They offer three puzzles. The first presents the image of an inn, The Two Crowns, and the following riddle:
“My name And sign is thirty Shillings just, and he that will tell My Name Shall have a Quart on trust, for why is not Five the Fourth Part of Twenty the Same in All Cases?”
This was easier to guess at the time of its inscription. The landlord of the Two Crowns was Mark Noble, the old English coin known as the noble was worth 6 shillings and eightpence, the mark was worth 13 shillings and fourpence, and two crowns were worth 10 shillings. Together these values total 30 shillings.
The second puzzle is more straightforward: “Subtract 45 From 45 That 45 May Remain.” This seems to refer to the following mathematical curiosity:
987654321 - 123456789 ----------- 864197532
Each of these figures comprises the digits 1 to 9, so all have the same digit sum: 45.
The last puzzle is the easiest:
AR
UBB
I
NGS
TONEF
ORAS
SE
S
Read this straight through and you get A RUBBING STONE FOR ASSES — possibly a comment by Francis on the loiterers who would gather outside his home.
The house was demolished in the 1960s, but the stones can be seen today in the foyer of the library at the Bebington civic center.
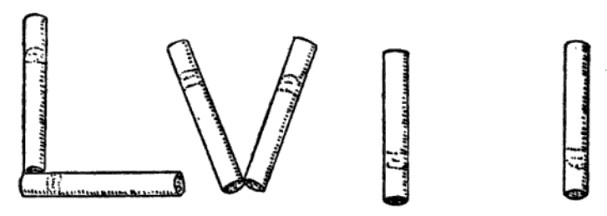
A puzzle by Henry Dudeney:
“It will be seen that we have arranged six cigarettes so as to represent the number 57. The puzzle is to remove any two of them you like (without disturbing any of the others) and so replace them as to represent 0, or nothing.”
A curious logic problem by Cambridge mathematician Max Newman, published in Hubert Phillips’ New Statesman puzzle column in 1933:
When Caliban’s will was opened it was found to contain the following clause:
‘I leave ten of my books to each of Low, Y.Y., and ‘Critic,’ who are to choose in a certain order:
- No person who has seen me in a green tie is to choose before Low.
- If Y.Y. was not in Oxford in 1920 the first chooser never lent me an umbrella.
- If Y.Y. or ‘Critic’ has second choice, ‘Critic’ comes before the one who first fell in love.’
Unfortunately, Low, Y.Y., and ‘Critic’ could not remember any of the relevant facts; but the family solicitor pointed out that, assuming the problem to be properly constructed (i.e., assuming it to contain no statement superfluous to its solution) the relevant data and order could be inferred. What was the prescribed order of choosing?
This does my head in — it’s a puzzle from the October 1958 issue of Eureka, the journal of the Cambridge University Mathematical Society:
“Below are shown the front elevation and plan of a mathematical figure. What is the side elevation?”

The terms (I believe) refer to multiview orthographic projection, the illustration technique used in architectural drawings: The front elevation is the view looking squarely at the “front” of the object, and the plan view looks down from above. What is the side view?
Add the same three letters, in order, both before and after the following to make a familiar English word:
ERGRO
A problem from the National Bank of New Zealand Competition 2000, via Crux Mathematicorum, November 2006:
Humanity is visited by three alien races, the Kweens, the Ozdaks, and the Merkuns. Kweens always speak the truth, and Ozdaks always lie. In any group of aliens, a Merkun never speaks first; when it does speak, it tells the truth if the previous statement was a lie and lies if the previous statement was truthful. The three alien races can tell one another apart, but to humans they all look the same. A delegation of three aliens visits Earth. At least one of them is a Kween. When they arrive they make the following statements, in order:
First alien: The second alien is a Merkun.
Second alien: The third alien is not a Merkun.
Third alien: The first alien is a Merkun.
Which aliens can we be sure are Kweens?