9 + 9 = 18; 9 × 9 = 81
24 + 3 = 27; 24 × 3 = 72
47 + 2 = 49; 47 × 2 = 94
497 + 2 = 499; 497 × 2 = 994
Science & Math
Two Milestones
The date 11/19/1999 contained only odd digits. Less than three months later, 2/2/2000 contained only even.
That’s a rare coincidence. It had been 1111 years since the last all-even date … and it’ll be 1111 more before the next all-odd one.
Skyward
In 1907, Massachusetts physician Duncan MacDougall conceived a singular experiment. When he observed that a patient at his Haverhill hospital was nearing death, he installed him in a specially constructed bed in his office and measured his weight both before and after death. With six such weighings he determined that humans lose between 0.5 and 1.5 ounces at death.
“Is the soul substance?” he wrote. “It would seem to me to be so. … Here we have experimental demonstration that a substance capable of being weighed does leave the human body at death.”
Similar experiments with 15 dogs showed no change in mass, proving, he decided, that dogs have no souls. MacDougall’s findings were written up briefly in the New York Times and occasioned a flurry of correspondence in American Medicine, but after that they were largely forgotten. But who knows? Perhaps he was right.
Math Notes
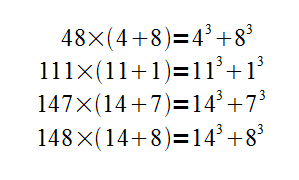
Pop Physics
A can of Diet Coke floats in water, while regular Coke sinks:
Why? The Diet Coke contains 190 mg of aspartame, but the regular Coke contains 39 grams of sugar. So the regular Coke is denser.
E Pluribus Unum
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111 111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
111111111111111111111111111111111111111111111111111111111111111111111111111111111111111111
11111111111111111111111111111111111111111 is prime.
Market Forces
The following question was a favourite topic for discussion, and thousands of the acutest logicians, through more than one century, never resolved it: ‘When a hog is carried to market with a rope tied about its neck, which is held at the other end by a man, whether is the hog carried to market by the rope or the man?’
— Isaac Disraeli, Curiosities of Literature, 1893
The Misfortune Field
One of the most enduring contributions to the [Wolfgang] Pauli legend was the ‘Pauli Effect,’ according to which Pauli could, by his mere presence, cause laboratory accidents and catastrophes of all kinds. Peierls informs us that there are well-documented instances of Pauli’s appearance in a laboratory causing machines to break down, vacuum systems to spring leaks, and glass apparatus to shatter. Pauli’s destructive spell became so powerful that he was credited with causing an explosion in a Göttingen laboratory the instant his train stopped at the Göttingen station.
– William H. Cropper, Great Physicists, 2004
(To exaggerate the effect, Pauli’s friends once arranged to have a chandelier crash to the floor when he arrived at a reception. When he appeared, a pulley jammed, and the chandelier refused to budge.)
The Mirror Problem
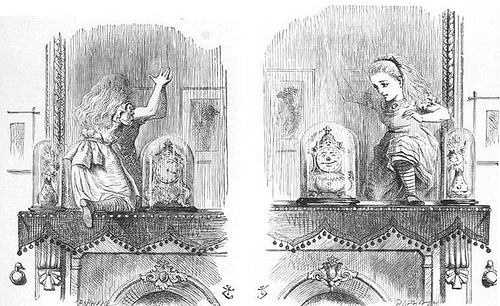
In 1868, 8-year-old Alice Raikes was playing with friends in her London garden when a visitor at a neighbor’s house overheard her name and called to her.
“So you are another Alice,” he said. “I’m very fond of Alices. Would you like to come and see something which is rather puzzling?” He led them into a room with a tall mirror in one corner.
‘Now,’ he said, giving me an orange, ‘first tell me which hand you have got that in.’ ‘The right,’ I said. ‘Now,’ he said, ‘go and stand before that glass, and tell me which hand the little girl you see there has got it in.’ After some perplexed contemplation, I said, ‘The left hand.’ ‘Exactly,’ he said, ‘and how do you explain that?’ I couldn’t explain it, but seeing that some solution was expected, I ventured, ‘If I was on the other side of the glass, wouldn’t the orange still be in my right hand?’ I can remember his laugh. ‘Well done, little Alice,’ he said. ‘The best answer I’ve had yet.’
“I heard no more then, but in after years was told that he said that had given him his first idea for Through the Looking-Glass, a copy of which, together with each of his other books, he regularly sent me.”
Bootstraps Everlasting
I recently visited an Eastern sage and asked him, ‘Is it possible to live for ever?’ ‘Certainly,’ he replied, ‘You must undertake to do two things.’ ‘What are they?’ ‘Firstly, you must never again make any false statements.’ ‘That’s simple enough. What is the second thing I must do?’ ‘Every day you must utter the statement “I will repeat this statement tomorrow.” If you follow these instructions faithfully you are certain to live forever.’
— Jacqueline Harman, letter to the Daily Telegraph, Oct. 8, 1985
