
A woman approached Bertrand Russell after a lecture.
“I’m so happy to find that you’re a solipsist,” she told him. “I’ve been one all my life, and I’m surprised there aren’t more of us.”

A woman approached Bertrand Russell after a lecture.
“I’m so happy to find that you’re a solipsist,” she told him. “I’ve been one all my life, and I’m surprised there aren’t more of us.”
There is a form of boat-racing, occasionally used at regattas, which affords a somewhat curious illustration of certain mechanical principles. The only thing supplied to the crew is a coil of rope, and they have, without leaving the boat, to propel it from one point to another as rapidly as possible. The motion is given by tying one end of the rope to the after thwart, and giving the other end a series of violent jerks in a direction parallel to the keel. I am told that in still water a pace of two or three miles an hour can be thus attained.
The chief cause for this result seems to be that the friction between the boat and the water retards all relative motion, but it is not great enough to affect materially motion caused by a sufficiently big impulse. Hence the usual movements of the crew in the boat do not sensibly move the centre of gravity of themselves and the boat, but this does not apply to an impulsive movement, and if the crew in making a jerk move their centre of gravity towards the bow n times more rapidly than it returns after the jerk, then the boat is impelled forwards at least n times more than backwards: hence on the whole the motion is forwards.
— W.W. Rouse Ball, Mathematical Recreations and Essays, 1905
2 × 5 × 27 = 1 × 15 × 18
2 + 5 + 27 = 1 + 15 + 18
213 × 624 = 312 × 426
102 × 402 = 201 × 204
936 × 213 = 639 × 312
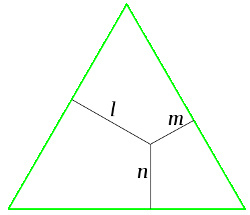
Pick any point inside an equilateral triangle and measure the distances to its sides.
The sum of those distances is the altitude of the triangle.
John Dalton was a tornado of English science, exploring atomic theory, meteorology, perception, and the physics of gases with equal avidity.
But he was a Quaker, and when in 1834 he was invited to be presented to William IV, the question arose whether he could properly appear in the scarlet robes of an Oxford doctor of laws, as the color was forbidden to him.
Dalton solved this neatly: He pointed out that he was color-blind. “You call it scarlet,” he said. “To me its color is that of nature — the color of green leaves.”
Published in 1869, Edward Everett Hale’s story “The Brick Moon” described the launch of an artificial satellite nearly a century before Sputnik:
If from the surface of the earth, by a gigantic peashooter, you could shoot a pea upward from Greenwich, aimed northward as well as upward; if you drove it so fast and far that when its power of ascent was exhausted, and it began to fall, it should clear the earth, and pass outside the North Pole; if you had given it sufficient power to get it half round the earth without touching, that pea would clear the earth forever. It would continue to rotate above the North Pole, above the Feejee Island place, above the South Pole and Greenwich, forever, with the impulse with which it had first cleared our atmosphere and attraction. If only we could see that pea as it revolved in that convenient orbit, then we could measure the longitude from that, as soon as we knew how high the orbit was, as well as if it were the ring of Saturn.
Because the 200-foot brick sphere is accidentally launched with human occupants, Hale perhaps also deserves credit for anticipating the space station.

Rutgers mathematician E.P. Starke posed this question in the American Mathematical Monthly of July 1940:
“In high school geometry texts and elsewhere one frequently meets the statement that the reason for the straightness of the crease in a folded piece of paper is that the intersection of two planes is a straight line. This is fallacious. What is the correct reason?”
I was going to post this as a puzzle, but after much pondering I’ve been unable to make sense of the answer. Here it is:
“Let P, P′ be two points of the paper that are brought into coincidence by the process of folding. Then any point A of the crease is equidistant from P, P′, since the lines AP, AP′ are pressed into coincidence. Hence the crease, being the locus of such points A, is the perpendicular bisector of PP′.”
I agree that this is true, but I don’t see what’s wrong with the first answer. Any ideas?
UPDATE: The consensus seems to be that the first answer makes some invalid assumptions, including flat planes and Euclidean space, where Starke’s proof is more rigorous. Thanks to everyone who’s written in.
(Second update, on reflection: Presumably the books that Starke mentions were not claiming that all creases must be straight, only that a straight crease is so because two planes intersect in a line. That still seems reasonable to me.)

A visitor to Niels Bohr’s cottage noticed a horseshoe nailed over the door.
“Surely you don’t expect that a horseshoe will bring good luck?” asked the visitor.
“No, I don’t,” Bohr said. “But they say it works even if you don’t believe in it.”
See The Misfortune Field.
What’s the key to this curious sequence of numbers?
1, 11, 21, 1211, 111221, 312211, 13112221, 1113213211, …
When read aloud, each term describes the one that precedes it. The first term consists of “one 1,” the second of “two 1s,” the third of “one 2, then one 1,” and so on.
That seems pretty arbitrary, but it opens a door into an unsuspected mathematical universe. Start with any number (except 22, an obvious dead end) and it will produce a string of digits that lengthens by about 30 percent with each generation — indeed, the percentage approaches a predictable constant (30.3577269 …) as the length approaches infinity.
More amazingly, the growing string will organize itself into a series of recognizable finite substrings that evolve predictably with each generation. John Horton Conway, who discovered all this, identified 92 such substrings, which he named after the chemical elements. Thus “uranium” (3) decays into “protactinium” (13), which becomes “thorium” (1113), and so on.
Thus an infinitely complex universe can arise from simply reading the number 1 aloud.
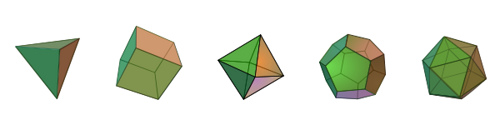
For any convex polyhedron,
vertices – edges + faces = 2
In 1988, readers of the Mathematical Intelligencer judged this the world’s second most beautiful theorem — behind Euler’s identity.