After seeing a manuscript of On the Origin of Species in April 1859, the Rev. Whitwell Elwin suggested that Darwin write about pigeons instead.
“This appears to me an admirable suggestion,” he wrote. “Everybody is interested in pigeons.”
After seeing a manuscript of On the Origin of Species in April 1859, the Rev. Whitwell Elwin suggested that Darwin write about pigeons instead.
“This appears to me an admirable suggestion,” he wrote. “Everybody is interested in pigeons.”
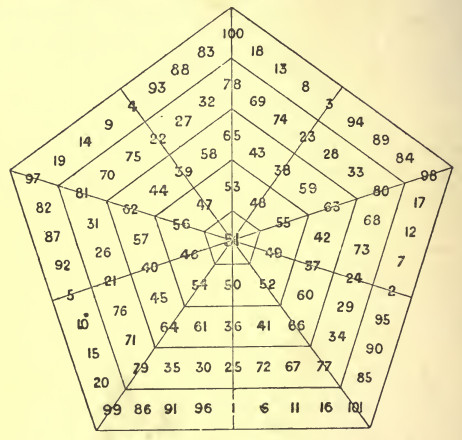
From Edward Falkener’s Games Ancient and Oriental and How to Play Them (1892), a magic pentagon. “It will be observed that the five sides of each pentagon are all equal, and that the five diameters, from one angle to the centre of the opposite side, are each 459, which is nine times the central number 51, which is also the mean number, the series being 1-101. And, further, that the inner pentagon is 510, or 10 times the mean number; the next pentagon 1,020, or 20 times the mean; the next 1,530, or 30 times the mean; and the outside pentagon 2,040, or 40 times the mean.” Evidently this was devised by Mikhail Frolov for Les carrés magiques (1886). (08/25/2015 Reader MJ has perfected this further, making each side of the outermost ring total 459, the same as the diagonals.)
From the Gentleman’s Magazine, October 1768, a “magic circle of circles” by Benjamin Franklin:
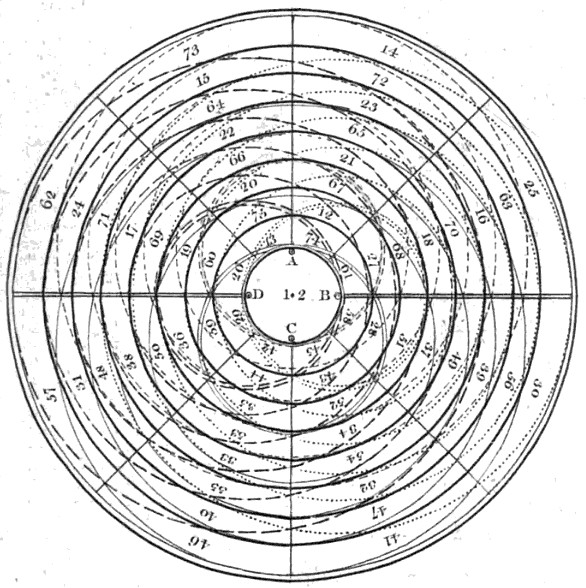
The numbers run from 12 to 75. Each ring and each radius, added to the central number 12, gives 360, the number of degrees in a circle. The dashed lines define four additional sets of circles, with centers at A, B, C, and D, each with five rings; each ring, when added to 12, also gives the total 360. (The 12 is added arbitrarily to bring the total to 360; remove it and the whole arrangement remains magic.)
From the inimitable R.V. Heath:
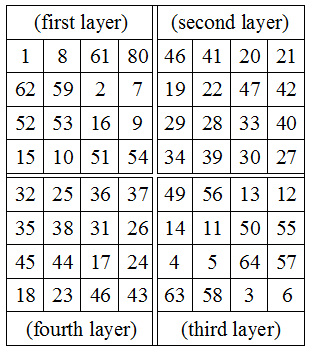
This 8×8 magic square can be cut into four smaller magic squares. When these four are stacked in the order indicated they produce a pandiagonal magic cube, each of whose rows, columns, and diagonals produces the total 130. Also: “The original eighth-order magic square has the additional property that if either set of alternate rows and either set of alternate columns be deleted — and this can be done in four ways — the remaining 16 numbers form a fourth-order magic square of magic constant 130.” (From Howard Whitley Eves, Mathematical Circles Squared, 1972.)
(Thanks, Ray.)

French anatomist Honoré Fragonard (1732-1799) blurred the line between science and art by arranging human and animal bodies in fanciful poses. By replacing the eyeballs with glass replicas and injecting a distorting resin into the facial blood vessels, he achieved some remarkably expressive effects — his Fetus Dancing the Jig is best left to the imagination.
Florence’s Museum of Zoology and Natural History preserves a collection of wax models that were used in teaching medicine in the 18th century (below). Modelers might refer to 200 corpses in preparing a single wax figure. “If we succeeded in reproducing in wax all the marvels of our animal machine,” wrote director Felice Fontana, “we would no longer need to conduct dissections, and students, physicians, surgeons and artists would be able to find their desired models in a permanent, odor-free and incorruptible state.” Goethe praised artificial anatomy as “a worthy surrogate that, ideally, substitutes reality by giving it a hand.”

(From Roberta Panzanelli, ed., Ephemeral Bodies, 2008.)
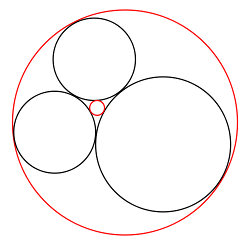
If three circles “kiss,” like the black circles above, then a fourth circle can be drawn that’s tangent to all three. In 1643 René Descartes showed that if the curvature or “bend” of a circle is defined as k = 1/r, then the radius of the fourth circle can be found by
![]()
The ± sign reflects the fact that two solutions are generally possible — the plus sign corresponds to the smaller red circle, the minus sign to the larger (circumscribing) one.
Frederick Soddy summed this up in a poem in Nature (June 20, 1936):
The Kiss Precise
For pairs of lips to kiss maybe
Involves no trigonometry.
‘Tis not so when four circles kiss
Each one the other three.
To bring this off the four must be
As three in one or one in three.
If one in three, beyond a doubt
Each gets three kisses from without.
If three in one, then is that one
Thrice kissed internally.
Four circles to the kissing come.
The smaller are the benter.
The bend is just the inverse of
The distance from the center.
Though their intrigue left Euclid dumb
There’s now no need for rule of thumb.
Since zero bend’s a dead straight line
And concave bends have minus sign,
The sum of the squares of all four bends
Is half the square of their sum.
To spy out spherical affairs
An oscular surveyor
Might find the task laborious,
The sphere is much the gayer,
And now besides the pair of pairs
A fifth sphere in the kissing shares.
Yet, signs and zero as before,
For each to kiss the other four
The square of the sum of all five bends
Is thrice the sum of their squares.
(Thanks, Sean.)
In January 1797 London tea broker James Tilly Matthews was committed to the Bethlem psychiatric hospital after increasingly erratic outbursts in which he claimed he was being persecuted by political enemies. In 1809 Matthews’ friends petitioned for his release, arguing that he was no longer insane, and Bethlem apothecary John Haslam published a book-length study showing how bad his case had become.
Matthews believed that a gang of spies were occupying a Roman wall near the asylum and torturing him with a device called an air loom. The loom was operated by “the Middle Man,” while “Sir Archy” and the “Glove Woman” focused its rays on Matthews and “Jack the Schoolmaster” recorded their effects. Similar gangs, Matthews said, were operating looms all over London to influence the thinking of the nation’s leaders. The tortures included “fluid locking,” “cutting soul from sense,” “stone making,” “thigh talking,” and “lobster-cracking,” which Matthews also described as “sudden death-squeezing”:
In short, I do not know any better way for a person to comprehend the general nature of such lobster-cracking operation, than by supposing himself in a sufficiently large pair of nut-crackers or lobster-crackers, with teeth which should pierce as well as press him through every particle within and without; he experiencing the whole stress, torture, driving, oppressing, and crush all together.
Matthews remained an asylum inpatient until his death in 1815. His is now considered to be the first documented case of paranoid schizophrenia.
The programming language Chef, devised by David Morgan-Mar, is designed to make programs look like cooking recipes. Variables are represented by “ingredients,” input comes from the “refrigerator,” output is sent to “baking dishes,” and so on. The language’s design principles state that “program recipes should not only generate valid output, but be easy to prepare and delicious,” but many of them fall short of that goal — one program for soufflé correctly prints the words “Hello world!”, but the recipe requires 32 zucchinis, 101 eggs, and 111 cups of oil to be combined in a bowl and served to a single person. Mike Worth set out to write a working program that could also be read as an actual recipe. Here’s what he came up with:
Hello World Cake with Chocolate sauce. This prints hello world, while being tastier than Hello World Souffle. The main chef makes a " world!" cake, which he puts in the baking dish. When he gets the sous chef to make the "Hello" chocolate sauce, it gets put into the baking dish and then the whole thing is printed when he refrigerates the sauce. When actually cooking, I'm interpreting the chocolate sauce baking dish to be separate from the cake one and Liquify to mean either melt or blend depending on context. Ingredients. 33 g chocolate chips 100 g butter 54 ml double cream 2 pinches baking powder 114 g sugar 111 ml beaten eggs 119 g flour 32 g cocoa powder 0 g cake mixture Cooking time: 25 minutes. Pre-heat oven to 180 degrees Celsius. Method. Put chocolate chips into the mixing bowl. Put butter into the mixing bowl. Put sugar into the mixing bowl. Put beaten eggs into the mixing bowl. Put flour into the mixing bowl. Put baking powder into the mixing bowl. Put cocoa powder into the mixing bowl. Stir the mixing bowl for 1 minute. Combine double cream into the mixing bowl. Stir the mixing bowl for 4 minutes. Liquify the contents of the mixing bowl. Pour contents of the mixing bowl into the baking dish. bake the cake mixture. Wait until baked. Serve with chocolate sauce. chocolate sauce. Ingredients. 111 g sugar 108 ml hot water 108 ml heated double cream 101 g dark chocolate 72 g milk chocolate Method. Clean the mixing bowl. Put sugar into the mixing bowl. Put hot water into the mixing bowl. Put heated double cream into the mixing bowl. dissolve the sugar. agitate the sugar until dissolved. Liquify the dark chocolate. Put dark chocolate into the mixing bowl. Liquify the milk chocolate. Put milk chocolate into the mixing bowl. Liquify contents of the mixing bowl. Pour contents of the mixing bowl into the baking dish. Refrigerate for 1 hour.
Worth confirmed that this correctly prints the words “Hello world!”, and then he used the same instructions to bake a real cake. “It was surprisingly well received,” he writes. “The cake was slightly dry (although nowhere near as dry as cheap supermarket cakes), but this was complimented well by the sauce. My brother even asked me for the recipe!”
While we’re at it: Fibonacci Numbers With Caramel Sauce.

In 1987, paleontologist Tom Rich was leading a dig at Dinosaur Cove southwest of Melbourne when student Helen Wilson asked him what reward she’d get if she found a dinosaur jaw. He said he’d give her a kilo (2.2 pounds) of chocolate. She did, and he did.
Encouraged, the students asked Rich what they’d get if they found a mammal bone. These are fairly rare among dinosaur fossils in Australia, so Rich rashly promised a cubic meter of chocolate — 35 cubic feet, or about a ton.
The cove was “dug out” by 1994, and paleontologists shut down the dig. Rich sent a curious unclassified bone, perhaps a turtle humerus, to two colleagues, who recognized it as belonging to an early echidna, or spiny anteater — a mammal.
Rich now owed the students $10,000 worth of chocolate. “It turns out that it is technically impossible to make a cubic meter of chocolate, because the center would never solidify,” he told National Geographic in 2005. So he arranged for a local Cadbury factory to make a cubic meter of cocoa butter, and then turned the students loose in a room full of chocolate bars.
“It was a bit like Willy Wonka,” Wilson said. “There were chocolate bars on the counters, the tables. We carried out boxes and boxes of chocolate.”
Fittingly, the new echidna was named Kryoryctes cadburyi.

In 1962 a Swedish motorist was fined for leaving his car too long in a space with a posted time limit. The motorist objected, saying that he had removed the car in time and then happened to return to the same spot later, resetting the time limit. The policeman defended his charge, saying that he had noted the positions of the valves on two of the tires — the front-wheel valve was in the 1 o’clock position, the rear-wheel valve at 8 o’clock. If the car had been moved, he argued, the valves were unlikely to take the same positions.
The court accepted the motorist’s claim, calculating that the chance that the valves would return to the same positions by chance was 1/12 × 1/12 = 1/144, great enough to establish reasonable doubt. The court added that if all four valves had been found to be in the same position, the lower likelihood (1/12 × 1/12 × 1/12 × 1/12 = 1/20,736, it figured) would have been enough to uphold the fine.
Is this right? In evaluating this reasoning, University of Chicago law professor Hans Zeisel notes that this method is biased in favor of the defendant, since the positions of the valves are not perfectly independent. He later added, “The use of the 1/144 figure for the probability of the constable’s observations on the assumption that the defendant had driven away also can be questioned. Not only may the rotations of the tires on different axles be correlated, but the figure overlooks the observation that the car was in the same parking spot. When a person leaves a parking place, it is far from certain that the spot will be available later and that the person will choose it again. For this reason, it has been said that the probability of a coincidence is even smaller than a probability involving only the valves.”
(Hans Zeisel, “Dr. Spock and the Case of the Vanishing Women Jurors,” University of Chicago Law Review, 37:1 [Autumn 1969], 1-18)
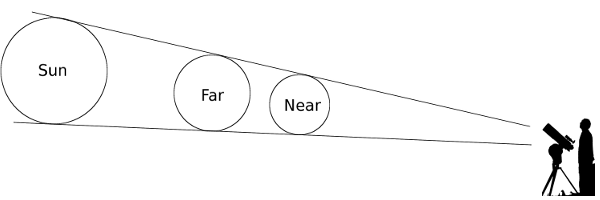
I am watching a double solar eclipse. The heavenly body Far, traveling east, passes before the sun. Beneath it passes the smaller body Near, traveling west. Far and Near appear to be the same size from my vantage point. Which do I see?
Common sense says that I see Near, since it’s closer. But Washington University philosopher Roy Sorensen argues that in fact I see Far. Near’s existence has no effect on the pattern of light that reaches my eyes. It’s not a cause of what I’m seeing; the view would be the same without it. (Imagine, for example, that Far were much larger and Near was lost in its shadow.)
“When objects are back-lit and are seen by virtue of their silhouettes, the principles of occlusion are reversed,” Sorensen concludes. “In back-lit conditions, I can hide a small suitcase by placing a large suitcase behind it.”
See In the Dark.
(Roy Sorensen, “Seeing Intersecting Eclipses,” Journal of Philosophy XCVI, 1 (1999): 25-49.)
In Other Inquisitions, Borges writes of a strange taxonomy in an ancient Chinese encyclopedia:
On those remote pages it is written that animals are divided into (a) those that belong to the Emperor, (b) embalmed ones, (c) those that are trained, (d) suckling pigs, (e) mermaids, (f) fabulous ones, (g), stray dogs, (h) those that are included in this classification, (i) those that tremble as if they were mad, (j) innumerable ones, (k) those drawn with a very fine camel’s hair brush, (l) others, (m) those that have just broken a flower vase, (n) those that resemble flies from a distance.
This is fanciful, but it has the ring of truth — different cultures can classify the world in surprisingly different ways. In traditional Dyirbal, an aboriginal language of Australia, each noun must be preceded by a variant of one of four words that classify all objects in the universe:
“The fact is that people around the world categorize things in ways that both boggle the Western mind and stump Western linguists and anthropologists,” writes UC-Berkeley linguist George Lakoff in Women, Fire, and Dangerous Things (1987). “More often than not, the linguist or anthropologist just throws up his hands and resorts to giving a list — a list that one would not be surprised to find in the writings of Borges.”