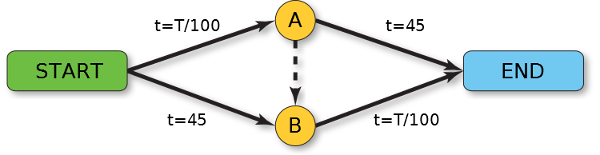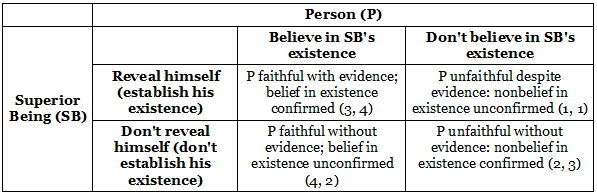
Is it rational to believe in the existence of a superior being? In 1982, New York University political scientist Steven J. Brams addressed the question using game theory. Assume that SB (the superior being) chooses whether to reveal himself, and P (a person) chooses whether to believe in SB’s existence. The two players have the following goals:
SB: Primary goal — wants P to believe in his existence. Secondary goal — prefers not to reveal himself.
P: Primary goal — wants belief (or nonbelief) in SB’s existence confirmed by evidence (or lack thereof). Secondary goal — prefers to believe in SB’s existence.
These goals determine the rankings of the four outcomes listed above. In each ordered pair, the first number refers to SB’s preference for that outcome (4 is high, 1 is low), and the second number refers to P’s preference. For example, SB prefers the two outcomes in which P believes in SB’s existence (because that’s his primary goal), and of these two outcomes, he prefers the one in which he doesn’t reveal himself (because that’s his secondary goal).
Brams finds a paradox here. If the game is one of complete information, then P knows that SB prefers not to reveal himself — that is, that SB prefers the second row to the first, regardless of P’s choice. And if SB will undoubtedly choose the second row, then P should choose his own preferred cell in that row, the second one. This makes (2, 3) the rational outcome of the game; it’s also the only outcome that neither player would choose unilaterally to depart once it’s chosen. And yet outcome (3, 4) would be preferred by both to (2, 3).
“Thus,” writes Brams, “not only is it rational for SB not to reveal himself and for P not to believe in his existence — a problem in itself for a theist if SB is God — but, more problematic for the rationalist, this outcome is unmistakably worse for both players than revelation by SB and belief by P, which would confirm P’s belief in SB’s existence.”
(Steven J. Brams, Superior Beings, 1983. This example is drawn largely from his paper “Belief in God: A Game-Theoretic Paradox,” in International Journal for Philosophy of Religion 13:3 [1982], 121-129.)