If we’re given 32 stones, each a different weight, how can we find both the heaviest and the second heaviest stone in 35 weighings with an equal-arm balance?
Puzzles
Black and White
The Dark Side

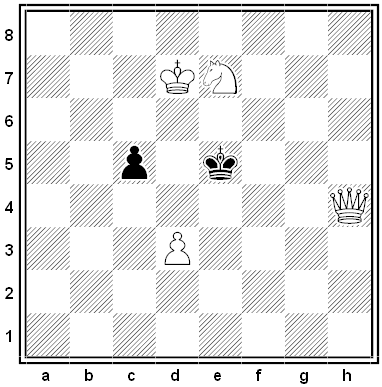
In a certain chess position, each row and each column contains an odd number of pieces. Prove that the total number of pieces on black squares is an even number.
Amnesia
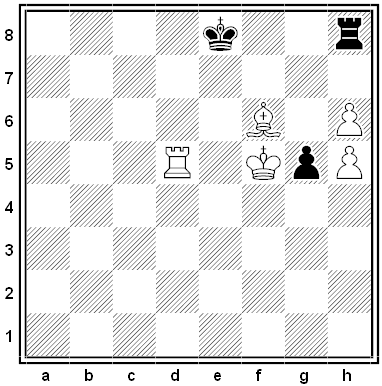
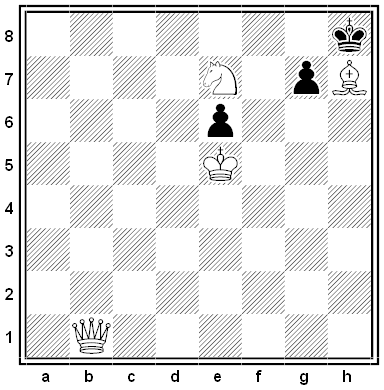
W. Langstaff offered this conundrum in Chess Amateur in 1922. White is to mate in two moves. He tries playing 1. Ke6, intending 2. Rd8#, but Black castles and no mate is possible. But by castling Black shows that his last move must have been g7-g5. Knowing this, White chooses 1. hxg6 e.p. rather than 1. Ke6. Now if Black castles he can play 2. h7#.
“Not so fast!” Black protests. “My last move was Rh7-h8, not g7-g5, so you can’t capture en passant.”
“Very well,” says White. “If you can’t castle, then I play 1. Ke6.” And we’re back where we started.
“What was really Black’s last move?” asks Burt Hochberg in Chess Braintwisters (1999). “If a position has a history, it can have only a single history, and Black would not be able to choose what his last move was any more than I can choose today what I had for dinner last night.”
“This is not a real game, however, but a problem in chess logic. The position’s history does not exist in actuality but only as a logical construct.”
Black and White
Hidden Sum
A problem from the 1973 American High School Mathematics Examination:
In this equation, each of the letters represents uniquely a different digit in base 10:
YE × ME = TTT.
What is E + M + T + Y?
Words and Numbers
If you write out the numbers from 1 to 5000 in American English (e.g., THREE THOUSAND EIGHT HUNDRED SEVENTY-THREE), it turns out that only one of them has a unique number of characters. Which is it? Spaces and hyphens count as characters.
“The Peace Problem”
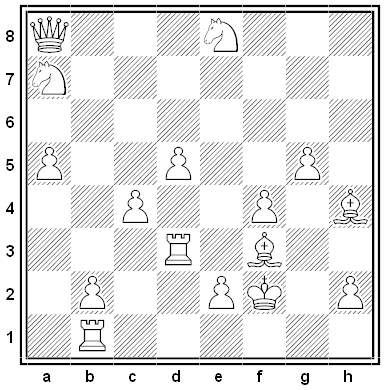
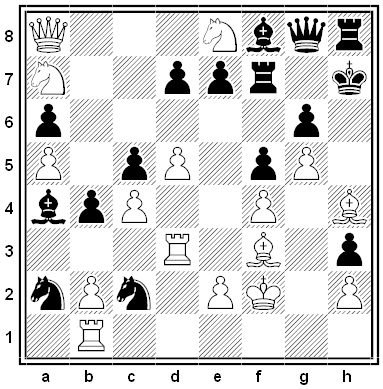
In 1984, at the height of the Cold War, Ukrainian chess journalist F.S. Bondarenko dedicated this puzzle to English chess editor A.J. Roycroft in the spirit of peace and goodwill.
White’s army is arranged as above. Add Black’s army (the standard complement of 8 pieces and 8 pawns) so that no piece of either color is under attack.
Quickie
From Crux Mathematicorum, May 1998:
If x is x% of y, and y is y% of z, where x, y, and z are positive real numbers, what is z?
Podcast Episode 86: Lateral Thinking Puzzles

Here are six new lateral thinking puzzles to test your wits and stump your friends — play along with us as we try to untangle some strange situations using only yes-or-no questions.