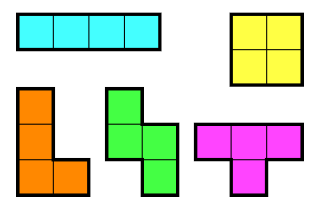
University of Waterloo mathematician Ross Honsberger chose this problem for his 2004 collection Mathematical Delights; it’s a generalization of a problem that Robert Gebhardt had offered in the Fall 1999 issue of Pi Mu Epsilon Journal. Paint the outside of an n × n × n cube red, then chop it into n3 unit cubes. Put the unit cubes in a box, mix them up thoroughly, withdraw one at random, and throw it across a table. What’s the probability that it comes to rest with a red face on top?
Puzzles
The Bishop Problem
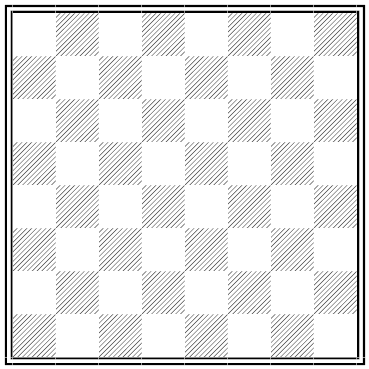
What is the minimum number of bishops needed to occupy or attack all squares on an 8 × 8 chessboard?
A Packing Problem
You need to pack for a midnight flight and the power is out. Your closet contains six pairs of shoes, six black socks, six gray socks, six pairs of brown gloves, and six pairs of tan gloves, but it’s too dark to distinguish colors or to match shoes. How many of each item must you take to be sure of having a matched pair of shoes, two socks of the same color, and a pair of matching gloves?
Black and White
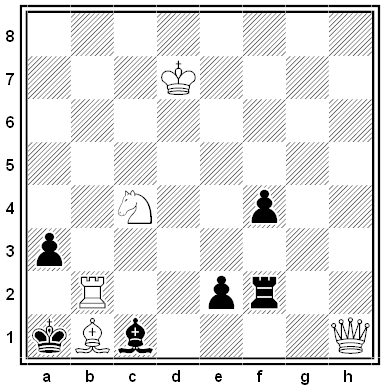
This problem, by G. Heathcote, won second prize in the Norwich Mercury‘s “King in the Corner” problem tournament, which required that Black’s king begin each problem in one of the board’s four corners. White to mate in two moves.
Egg Hunt
A puzzle by Tim C., an applied research mathematician at the National Security Agency, from the agency’s January 2017 Puzzle Periodical:
Alice has a dozen cartons, arranged in a 3×4 grid, which for convenience we have labeled A through L:

She has randomly chosen two of the cartons and hidden an Easter egg inside each of them, leaving the remaining ten cartons empty. She gives the dozen cartons to Bob, who opens them in the order A, B, C, D, E, F, G, H, I, J, K, L until he finds one of the Easter eggs, whereupon he stops. The number of cartons that he opens is his score. Alice then reseals the cartons, keeping the eggs where they are, and presents the cartons to Chris, who opens the cartons in the order A, E, I, B, F, J, C, G, K, D, H, L, again stopping as soon as one of the Easter eggs is found, and scoring the number of opened cartons. Whoever scores lower wins the game; if they score the same then it’s a tie.
For example, suppose Alice hides the Easter eggs in cartons H and K. Then Bob will stop after reaching the egg in carton H and will score 8, while Chris will stop after reaching the egg in carton K and will score 9. So Bob wins in this case.
Who is more likely to win this game, Bob or Chris? Or are they equally likely to win?
Throughput
A bedeviling little puzzle from Dickinson College mathematician David Richeson.
A topologist, it is said, is someone who can’t tell his donut from his coffee mug.
Double Duty
On Nov. 5, 1996, Election Day in the United States, the New York Times crossword puzzle carried a surprising clue:
39. Lead story in tomorrow’s newspaper (!), with 43A
43 across turned out to be ELECTED, but 39 across might be either CLINTON or BOBDOLE — both possibilities had seven letters. Was the Times venturing to guess the outcome of the day’s election?
No. Composer Jeremiah Farrell had contrived each of the seven down clues to admit of two possible answers, so that no matter which candidate won, the newspaper might claim a “correct” result.
Crossword editor Will Shortz called Farrell’s ambiguous effort his favorite puzzle of all time.
(Thanks, Andrew.)
Moving Day
Black and White
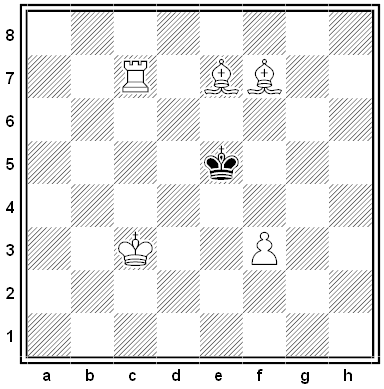
A problem “for juvenile solvers” by Edith Baird, from Womanhood, May 1899. White to mate in two moves.
A Confusing Country
Philosopher Nelson Goodman published this puzzle anonymously in the Boston Post in 1931, at age 24. He later called it “by far the most popular and widely circulated of all my writings.”
All the men of a certain country are either nobles or hunters, and no one is both a noble and a hunter. The male inhabitants are so nearly alike that it is difficult to tell them apart, but there is one difference: nobles never lie, and hunters never tell the truth.
Three of the men meet one day and Ahmed, the first, says something. He says either, ‘I am a noble’, or ‘I am a hunter.’ (We don’t know yet which he said.)
Ali, the second man, heard what Ahmed said, and in reply to a query, answered, ‘Ahmed said, “I am a hunter”.’ Then Ali went on to say, ‘Azab is a hunter.’
Azab was the third man. He said, ‘Ahmed is a noble.’
Now the problem is, which is each? How do you know?