Mount Everest rises 29,029 feet above sea level, and Ecuador’s volcano Chimborazo rises only 20,702 feet. But because Earth bulges at the equator, Chimborazo is actually farther from the center of the planet. If we could connect the two peaks with a water pipe, in which direction would the water flow?
Puzzles
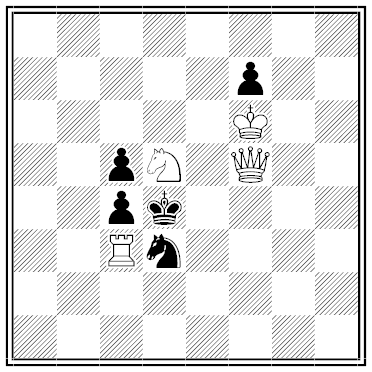
Black and White
Spin City
Imagine two concentric roulette wheels, each divided into 100 sectors. Choose 50 sectors at random on each wheel, paint them black, and paint the rest white. Prove that we can now position the wheels so that at least 50 of the aligned sectors match.
Horse Play
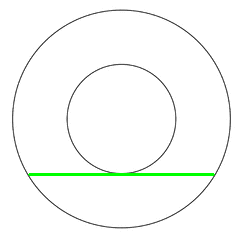
A carnival worker is asked to paint the deck of a carousel. Because the center of the carousel is occupied by machinery, he can’t measure its diameter or even its radius. The best he can do is to take the measurement shown in green, which is 42 feet.
He’s explaining this apologetically when his supervisor stops him. “That’s all the information we need,” he says. “That’s enough to tell us how much paint to buy.”
How did they go about it?
Leap Day
Alice gets a rocket-powered pogo stick for her birthday. She jumps 1 foot on the first hop, 2 feet on the second, then 4, 8, and so on. This gets alarming. By judicious hopping, can she arrange to return to her starting point?
Desperate Measuring

You’re alone on a desert island and want to lay out a course for some snail races. Unfortunately, you have only an 8.5 x 11 inch sheet of paper. How can you use it to measure exactly 3 inches?
The Last Cent

You and a friend are playing a game. Between you is a pile of 15 pennies. You’ll take turns removing pennies from the pile — each of you, on his turn, can choose to remove 1, 2, or 3 pennies. The loser is the one who removes the last penny.
You go first. How should you play?
Fish Story
I release a fish at the edge of a circular pool. It swims 80 feet in a straight line and bumps into the wall. It turns 90 degrees, swims another 60 feet, and hits the wall again. How wide is the pool?
Black and White
Changing Colors

Fifty-five chameleons live on a tropical island. Thirteen are green, 19 are brown, and 23 are gray. Whenever two chameleons of different colors meet, both change to the third color. Is it possible that all 55 chameleons might eventually be the same color?