Puzzles
Sand Reckoning
Using only a 4-minute hourglass and a 7-minute hourglass, how can you measure 9 minutes?
Spin Control

We’re playing Russian roulette. The revolver has six chambers, all empty. I put bullets in two adjacent chambers, spin the cylinder, hold the gun to my head, and pull the trigger. It clicks. Now it’s your turn. Before pulling the trigger, you can choose to spin the cylinder again or leave it as it is. Which is better?
Secret Entrance
Puzzle maven Noboyuki Yoshigahara noticed this sign on the glass door of an Osaka restaurant:

What is its meaning?
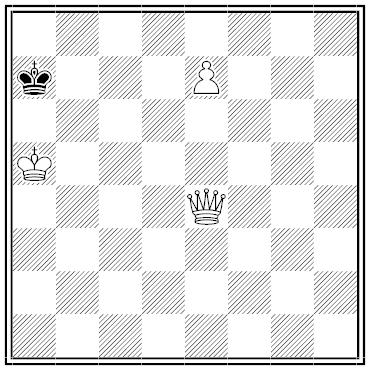
Black and White
Making Way

A man is 4/7 across a train trestle when he sees a train coming. To get off the trestle, he can run toward the train or away from it. As it happens, in either case he’ll reach safety just as the locomotive passes him. If he runs at 20 kph, how fast is the train going?
Strictly Speaking
Six logicians finish dinner. The waitress asks, “Do you all want coffee?”
First logician: “I don’t know.”
Second logician: “I don’t know.”
Third logician: “I don’t know.”
Fourth logician: “I don’t know.”
Fifth logician: “I don’t know.”
Sixth logician: “No.”
Who gets coffee and why?
Good Hands
A surgeon wears sterile gloves both to protect his patient and to protect himself. Can a hard-pressed surgeon with only two pairs of sterile gloves operate on three patients while keeping everyone safe?
War Games

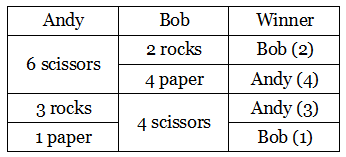
Andy and Bob play rock-paper-scissors 10 times. Andy plays 3 rocks, 6 scissors, and 1 paper (in some order), and Bob plays 2 rocks, 4 scissors, and 4 paper. There are no ties. Who wins?
Black and White
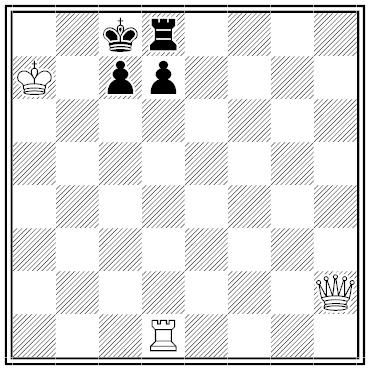
Future world chess champion Max Euwe composed this miniature in 1927. White to mate in two moves.