If you were a British soldier in Malta in the 19th century, you might receive this card from a local dram-shop:

What does it mean?
If you were a British soldier in Malta in the 19th century, you might receive this card from a local dram-shop:

What does it mean?

From Amusements in Mathematics by Henry Ernest Dudeney (1917):
The men in the illustration are disputing over the liquid contents of a barrel. What the particular liquid is it is impossible to say, for we are unable to look into the barrel; so we will call it water. One man says that the barrel is more than half full, while the other insists that it is not half full. What is their easiest way of settling the point? It is not necessary to use stick, string, or implement of any kind for measuring. I give this merely as one of the simplest possible examples of the value of ordinary sagacity in the solving of puzzles. What are apparently very difficult problems may frequently be solved in a similarly easy manner if we only use a little common sense.
Okay, I’ll ask three questions, and if you miss one I get your house. Fair enough? Here we go:
Don’t be hasty — your house is on the line.
This question was proposed in the Scientific American, in 1868: ‘How many revolutions upon its own axis, will a wheel make in rolling once around a fixed wheel of the same size?’
The question brought to the editor of that paper many replies all claiming to have solved it. Yet the replies were about equally divided as to the number of revolutions, one part claiming one revolution and the other two revolutions. So much interest was manifested in it that Munn & Co. published The Wheel, June, 1868. It contains 72 pages, giving many of the solutions, illustrated by many diagrams.
— Miscellaneous Notes and Queries, August 1889
So who’s right?
You’re in a rowboat in a swimming pool, and you’re holding a cannonball. If you throw the ball into the pool, will the water level rise or fall?

Three beautiful women and their jealous husbands want to cross a river, but the boat will hold only two people at a time. How can they arrange the crossing if no woman is to remain with a man unless her husband is present?
The knight’s tour is a recreation familiar to chessplayers: Move a knight about an empty chessboard so as to visit each square exactly once.
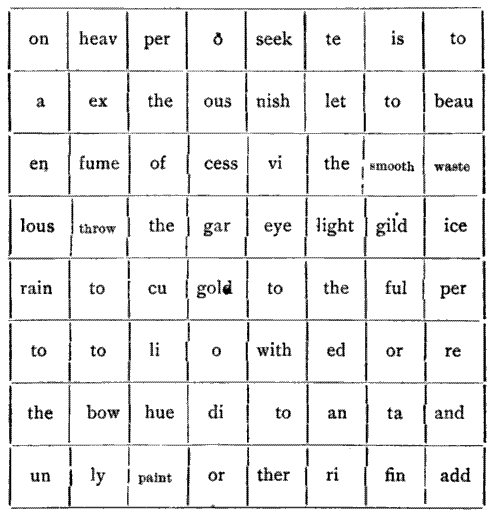
On this board, each square contains a syllable. Collect them in the right order and you’ll compose a six-line quotation from Shakespeare. What is it?
(Hint: Start on e4, “to”.)
Take an ordinary chessboard and cut off two diagonally opposite corners. Now: Is it possible to tile the remaining 62 squares with 31 dominoes?
This calls for inspiration rather than trial and error. Most people see the solution immediately or not at all.
First published in 1671, this anonymous verse came with a simple instruction that would render it into sense. Can you discover it?
I saw a peacock with a fiery tail
I saw a blazing comet drop down hail
I saw a cloud with ivy circled round
I saw a sturdy oak creep on the ground
I saw a pismire swallow up a whale
I saw a raging sea brim full of ale
I saw a venice glass sixteen foot deep
I saw a well full of men’s tears that weep
I saw their eyes all in a flame of fire
I saw a house as big as the moon and higher
I saw the sun even in the midst of night
I saw the man that saw this wondrous sight.
I saw a pack of cards gnawing a bone
I saw a dog seated on Britain’s throne
I saw King George shut up within a box
I saw an orange driving a fat ox
I saw a butcher not a twelvemonth old
I saw a great-coat all of solid gold
I saw two buttons telling of their dreams
I saw my friends who wished I’d quite these themes.
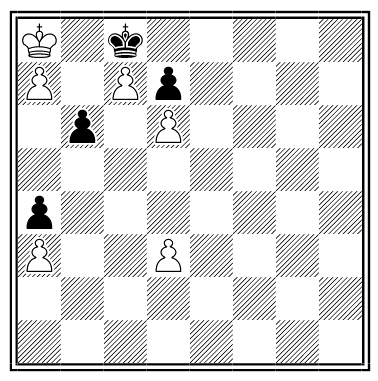
White to move. I won’t give the solution — try it out and you’ll see why.
(Composed by V. Röpke, Skakbladet, 1942.)