French mathematician Joseph Bertrand offered this observation in his Calcul des probabilités (1889). Inscribe an equilateral triangle in a circle, and then choose a chord of the circle at random. What is the probability that this chord is longer than a side of the triangle? There seem to be three different answers:

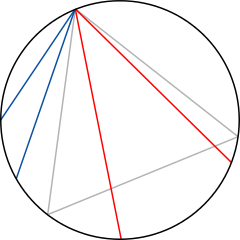
1. Choose two random points on the circle and join them, then rotate the triangle until one of its vertices coincides with one of these points. Now the chord is longer than a side of the triangle when its farther end falls on the arc between the other two vertices of the triangle. That arc is one third of the total circumference of the circle, so by this argument the probability is 1/3.

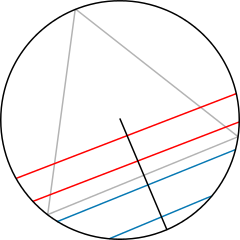
2. Choose a radius of the circle, choose a point on that radius, and draw a chord through that point that’s perpendicular to the radius. Now imagine rotating the triangle so that one of its sides also intersects the radius perpendicularly. Our chord will be longer than a side of the triangle if the point we chose is closer to the circle’s center than the point where the triangle’s side intersects the radius. The triangle’s side bisects the radius, so by this argument the probability is 1/2.

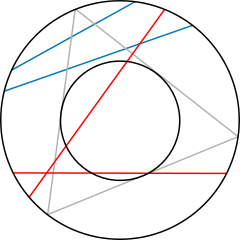
3. Choose a point anywhere in the circle and draw the chord for which this is the midpoint. This chord will be longer than a side of the triangle if the point we chose falls within a concentric circle whose radius is half the radius of the larger circle. That smaller circle has one-fourth the area of the larger circle, so by this argument the probability is 1/4.

Further methods yield still further solutions. After more than a century, the implications of Bertrand’s conundrum are still being discussed.