
“A man who has not read Homer is like a man who has not seen the ocean. There is a great object of which he has no idea.” — Walter Bagehot

“A man who has not read Homer is like a man who has not seen the ocean. There is a great object of which he has no idea.” — Walter Bagehot
Proverbs from around the world:

The Chief Defect of Henry King
Was chewing little bits of String.
At last he swallowed some which tied
Itself in ugly Knots inside.
Physicians of the Utmost Fame
Were called at once; but when they came
They answered, as they took their Fees,
“There is no Cure for this Disease.
“Henry will very soon be dead.”
His Parents stood about his Bed
Lamenting his Untimely Death,
When Henry, with his Latest Breath,
Cried, “Oh, my Friends, be warned by me,
That Breakfast, Dinner, Lunch, and Tea
Are all the Human Frame requires …”
With that, the Wretched Child expires.
— Hilaire Belloc
Writing in the Wall Street Journal about long sentences in literature, Laurie Winer offered her thoughts in a single sentence of 854 words:
In order to credit William Faulkner — as the Guinness Book of World Records does — with the longest sentence in literature, one must include, when counting the words in Faulkner’s erratically punctuated, loosely defined ‘sentences,’ lengthy italicized passages that echo what passes through a character’s mind; not to mention parentheses within parentheses; and long sentences connected to sentence fragments by dashes where periods, strictly speaking, should be; as well as run-on paragraphs that begin audaciously with a lower-case letter; and, while Guinness says the longest sentence is a 1,300-word tirade in Absalom, Absalom! there is, by liberal Faulknerian standards, a 1,928-word sentence (beginning ‘They both bore it as though in deliberate flagellant exaltation’) in that book which contains a 1,360-word parenthetical memory/thought that has within it at least 32 traditional sentences; so perhaps Faulkner should not be holding this title after all (from his London office a Guinness editor, Colin Smith, who says he has never seen Absalom, Absalom!, names as his source for the longest sentence entry the 1945 Bookmen’s Bedlam of Literary Oddities, a fustian collection of curiosa by bibliophile Walter Hart Blumenthal, which doesn’t mention Faulkner at all and instead gives the palm for sequential verbosity to Edward Phillips’s Preface to Theatrum Poetarium, written in 1675, for a 1,012-word sentence) even though others, including the writer Malcolm Cowley, cite another Faulkner sentence, found in the story ‘The Bear,’ as among the longest ever written; viz., in his introduction to the story in the 1946 The Portable Faulkner, Mr. Cowley calls this whopper, which begins ‘To him it was as though the ledgers in their scarred cracked leather bindings,’ a 1,800-word sentence when in fact it is, by the most liberal definition, a 1,600-word Faulknerian sentence, which is, under closer scrutiny, a 91-word sentence with no period followed by a new paragraph (indented) beginning with a lower-case letter that contains nothing but a 67-word sentence fragment that is followed by another paragraph fragment, etc. (even Albert Erskine, Faulkner’s editor at Random House, says of the long word group in ‘The Bear’: ‘New sentences begin whether the author puts a period there or not’), which may sound petty, but, if you’re going to call something the longest sentence, the term sentence must have some meaning or else what’s the point of bestowing the title (you may believe, as a confident New York City librarian told me, that the longest sentence in literature is the last 40,000 words of James Joyce’s Ulysses, which does contain two periods but which is really a poem, a chant, or free association that disintegrates at times to a point where it is unrecognisable as formal grammar or even as English (‘… I can see his face clean shaven Frseeeeeeeeeeeeeeeeeeeeefrong that train …’) and clings only, as the critic Roy K. Gottfried points out, to a morphemic structure, though while Joyce broke ground and freed his prose from the tyranny of syntax, he did not write a 40,000-word sentence) unless you give it to someone who actually wrote an extremely long sentence, as Marcel Proust did in his seven-volume masterpiece (first published as eight volumes, though Bookmen’s Bedlam calls it eleven and another reference book, Felton & Fowler’s Best, Worst, and Most Unusual, remembers it as sixteen — these record books seem to get none of the numbers right) Remembrance of Things Past, which contains in it a famous, perfect 958-word (in the C.K. Scott Moncrieff translation) sentence (it begins ‘Their honour precarious, their liberty provisional …’) that appears near the start of the fourth book, Sodome et Gomorrhe or, as it is known in English, Cities of the Plain, just after the narrator has witnessed a homosexual encounter between Jupien the tailor and the Baron de Charlus, an encounter that initiates a rumination on the part of our young hero, whose creator was himself a half-Jewish homosexual, on the tenuous situation of the homosexual in society and on how he is like the Jew in respect to the duplicity of his life, seeking to assimilate and yet compelled to remain different, permeated with the pain of the ever-present knowledge that, because of what he is, he gives cause to others to snub him, alienate him, or hate him, and of how this difference, shared by members on the highest and lowest rungs of society, bonds the ambassador to the felon, or the prince to the ruffian, for here is a sentence that does not suffocate the reader with its verbiage (as might the work of certain Teutotonic runners-up for the longest sentence, such as Thomas Bernhard or Hermann Broch); here is a sentence whose length befits its subject matter and its context in the whole; here is a sentence that can be parsed; here is a sentence that should be called the longest in literature (taking into account the possibility that there exist longer grammatical sentences — maybe some crank somewhere wrote a one-sentence book — but we are biased in favor of our titleholder’s also being a genius) by the Guinness people; so we suggest they change their Faulkner entry.
She followed this with a one-word paragraph: “Now.”
(Via Willard R. Espy’s The Word’s Gotten Out, 1989.)
On hearing that Watership Down was a novel about rabbits written by a civil servant, Craig Brown wrote, “I would rather read a novel about civil servants written by a rabbit.”
I stole through the dungeons, while everyone slept,
Till I came to the cage where the Monster was kept.
There, locked in the arms of a Giant Baboon,
Rigid and smiling, lay … Mrs. RAVOON!
I climbed the clock-tower in the first morning sun
And ’twas midday at least ere my journey was done;
But the clock never sounded the last stroke of noon,
For there, from the clapper, swung Mrs. RAVOON.
I ran through the marsh ‘midst the lightning and thunder,
When a terrible flash split the darkness asunder.
Chewing a rat’s tail and mumbling a rune,
Mad in the moat, squatted Mrs. RAVOON.
I stood by the waters so green and so thick,
And I stirred at the scum with my old, withered stick;
When there rose through the ooze, like a monstrous balloon,
The bloated cadaver of Mrs. RAVOON.
I hauled in the line, and I took my first look
At the half-eaten horror that hung from the hook.
I had dragged from the depths of the limpid lagoon
The luminous body of Mrs. RAVOON.
Facing the fens, I looked back from the shore
Where all had been empty a moment before;
And there, by the light of the Lincolnshire moon,
Immense on the marshes, stood … Mrs. RAVOON!
After this memorable debut in For Love and Money (1956), Paul Dehn’s macabre character has found her way into songs and poems by many writers, even beyond her creator’s death in 1976. They’re cataloged here.
“Lady Dillon told Sir F. Chantrey that English women were more buxom than Italian women. The delicate way she put it was, ‘you will find that Italian women can sit much closer to a wall than English.'”
— George Lyttelton’s Commonplace Book, 2002
A surprising detail from Duke Ellington’s childhood, from his 1973 autobiography Music Is My Mistress:
There were many open lots around Washington then, and we used to play baseball at an old tennis court on Sixteenth Street. President Roosevelt would come by on his horse sometimes, and stop and watch us play. When he got ready to go, he would wave and we would wave at him. That was Teddy Roosevelt — just him and his horse, nobody guarding him.
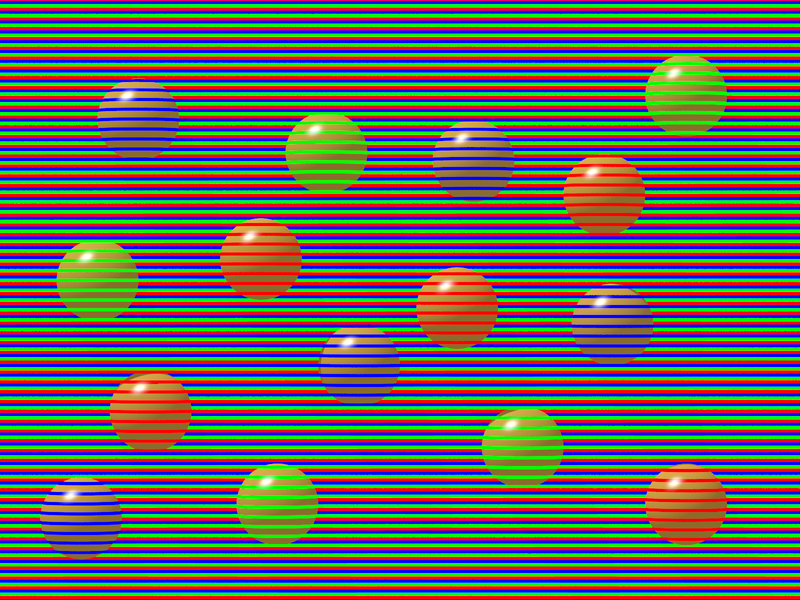
An illusion by University of Texas engineer David Novick: All the spheres have the same light-brown base color (RGB 255,188,144). The intervening foreground stripes seem to impart different hues. See this Twitter thread for the same image with the foreground stripes removed.
Have the love and fear of God ever before thine eyes; God confirm your faith in Christ and that you may live accordingly, Je vous recommende a Dieu. If you meet with any pretty insects of any kind keep them in a box.
— Sir Thomas Browne, letter to his son, 1661