Any pair of points define an infinity of ellipses and an infinity of hyperbolas.
The ellipses do not touch one another, nor do the hyperbolas.
But every ellipse meets every hyperbola at a right angle.
Dedication
There was many years ago a Lazy Man’s Society organised in Manchester. One of the articles required that no man belonging to it should ever be in a hurry. Should he violate this article he must stand treat to the other members. Now, it happened once on a time that a doctor was driving post-haste through the streets to visit a patient. The members of the society saw him and chuckled over the idea of a treat, and on his return reminded him of his fast driving and violation of the rules. ‘Not at all,’ said the doctor. ‘The truth is, my horse was determined to go, and I felt too lazy to stop him.’ They did not catch him that time.
— Tit-Bits From All the Most Interesting Books, Periodicals, and Newspapers in the World, Oct. 22, 1881
“Bonaparte and the Echo”
Bonaparte: Alone I am in this sequestered spot, not overheard.
Echo: Heard.
Bonaparte: ‘Sdeath! Who answers me? What being is there nigh?
Echo: I.
Bonaparte: Now I guess! To report my accents Echo has made her task.
Echo: Ask.
Bonaparte: Knowest thou whether London will henceforth continue to resist?
Echo: Resist.
Bonaparte: Whether Vienna and other courts will oppose me always?
Echo: Always.
Bonaparte: O, Heaven! what must I expect after so many reverses?
Echo: Reverses.
Bonaparte: What! should I, like a coward vile, to compound be reduced?
Echo: Reduced.
Bonaparte: After so many bright exploits be forced to restitution?
Echo: Restitution.
Bonaparte: Restitution of what I’ve got by true heroic feats and martial address?
Echo: Yes.
Bonaparte: What will be the fate of so much toil and trouble?
Echo: Trouble.
Bonaparte: What will become of my people, already too unhappy?
Echo: Happy.
Bonaparte: What should I then be that I think myself immortal?
Echo: Mortal.
Bonaparte: The whole world is filled with the glory of my name, you know.
Echo: No.
Bonaparte: Formerly its fame struck this vast globe with terror.
Echo: Error.
Bonaparte: Sad Echo, begone! I grow infuriate! I die!
Echo: Die!
It’s said that the Nuremberg bookseller who penned this clever bit of sedition was court-martialed and shot in 1807. Napoleon later said, “I believe he met with a fair trial.”
The Black Hole
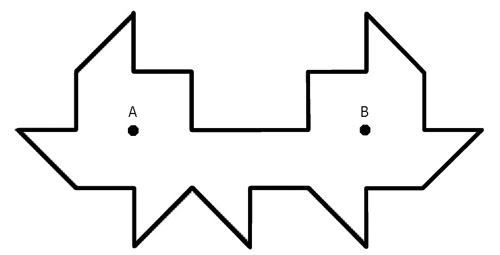
If Satan plays miniature golf, this is his favorite hole. A ball struck at A, in any direction, will never find the hole at B — even if it bounces forever.
The idea arose in the 1950s, when Ernst Straus wondered whether a room lined with mirrors would always be illuminated completely by a single match.
Straus’ question went unanswered until 1995, when George Tokarsky found a 26-sided room with a “dark” spot; two years later D. Castro offered the 24-sided improvement above. If a candle is placed at A, and you’re standing at B, you won’t see its reflection anywhere around you — even though you’re surrounded by mirrors.
“Singular Intermarriages”
There were married at Durham, Canada East, an old lady and gentleman, involving the following interesting connections:–
The old gentleman is married to his daughter’s husband’s mother-in-law, and his daughter’s husband’s wife’s mother. And yet she is not his daughter’s mother; but she is his grandchildren’s grandmother, and his wife’s grandchildren are his daughter’s step-children. Consequently the old lady is united in the bonds of holy matrimony and conjugal affection to her daughter’s brother-in-law’s father-in-law, and her great-grandchildren’s grandmother’s step-father; so that her son-in-law may say to his children, Your grandmother is married to my father-in-law, and yet he is not your grandfather; but he is your grandmother’s son-in-law’s wife’s father. This gentleman married his son-in-law’s father-in-law’s wife, and he is bound to support and protect her for life. His wife is his son-in-law’s children’s grandmother, and his son-in-law’s grandchildren’s great-grandmother.
— Charles Carroll Bombaugh, Gleanings for the Curious from the Harvest-fields of Literature, 1875
The Franklin Square
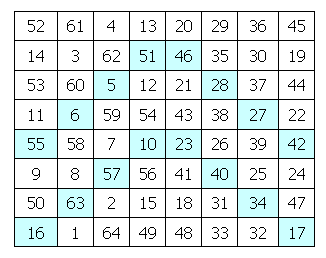
In a 1769 letter, Ben Franklin describes a magic square he devised in his youth. The magic total of 260 can be reached by adding the numbers in each row or column, as in a normal magic square. But “bent rows” (shaded) produce the same total, even when “wrapped across” the border of the table. This works in all four directions.
Further: Half of each row or column sums to half of 260, as does any 2×2 subsquare. And the four corners and the four center squares sum to 260. (Alas, the main diagonals don’t, so this doesn’t strictly qualify as a magic square by the modern definition.)
Interestingly, no one knows how Franklin created the square. Many methods have been devised, but none apparently as quick as his, which he claimed could generate them “as fast as he could write.”
“Singular Discovery at Aberdeen”
While in course of demolishing a block of old houses on the north side of Longacre, which requires to be removed for the extension of Marischal College buildings, the workmen made a curious discovery yesterday morning. About fifteen inches from the exterior of a wall composed of solid masonry they came across a couple of crabs, one being dead but still in a fresh state, and the other alive, although so attenuated as to be almost transparent. The crabs were handed over to Mr. Jones, assistant professor of chemistry at Marischal College. The live crab is preserved in a jar containing water. In size it is an inch long and a quarter broad, its dead companion being an inch and three-quarters in length and an inch in breadth. The house has been untenanted for six months, and it is a mystery how the creatures could have found their way into a mass of masonry twenty feet above the ground level of the outside, and three or four feet from the level of the floor.
— Newspaper paragraph quoted in Scottish Notes and Queries, February 1896
The Perfect Family
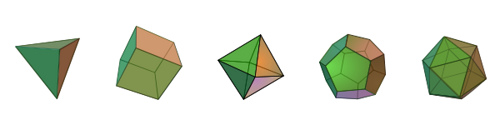
Take any Platonic solid, join the centers of its faces, and, charmingly, you get another Platonic solid. The cube and the octahedron produce one another, as do the dodecahedron and the icosahedron, and the tetrahedron produces another tetrahedron.
Bonus factoid: If you inscribe a dodecahedron and an icosahedron in the same sphere, the dodecahedron will occupy more of the sphere’s volume. It has fewer faces than the icosahedron, but its faces are more nearly circular, so it fits the sphere more snugly.
See The Pup Tent Problem.
In a Word
happify
v. to make happy
All God’s Creatures
Ernest Thompson Seton (1860-1946) loved nature and loved God — so in a 1907 book he tried to prove that animals follow the 10 commandments:
- Thou shalt not steal: “A stick found in the woods is the property of the Rook that discovers it, and doubly his when he has labored to bring it to his nest. This is recognized law.”
- Thou shalt not kill: “New born Rattlesnakes will strike instantly at a stranger of any other species, but never at one of their own.”
- Honor thy father and mother: “A Hen sets out with her Chickens a-foraging; one loiters, does not hasten up at her ‘cluck cluck’ of invitation and command; consequently he gets lost and dies.”
- Thou shalt not commit adultery: “The promiscuous animals to-day–the Northwestern Rabbit and the Voles–are high in the scale of fecundity, low in the scale of general development, and are periodically scourged by epidemic plagues.”
- Thou shalt not bear false witness: “Oftentimes a very young Hound will jump at a conclusion, think, or hope, he has the trail, then allowing his enthusiasm to carry him away, give the first tongue, shouting in Hound language, ‘Trail!’ The other Hounds run to this, but if a careful examination shows that he was wrong, the announcer suffers in the opinion of the pack, and after a few such blunders, that individual is entirely discredited.”
- Thou shalt not covet: “A Hen had made a nest in a certain place, and was already sitting. Later another Hen, desiring the same nest, took possession several times during the owner’s brief absence, adding some of her own eggs, and endeavoring to sit. The result was a state of war, and the eggs of both Hens were destroyed.”
Actually, he runs out of gas here — Seton was unable to convince even himself that animals avoid making graven images, swearing, or working on Sunday. So he concludes The Natural History of the Ten Commandments by deciding that “Man is concerned with all” the commandments, “the animals only with the last six.”