A problem from the Tenth International Mathematical Olympiad, 1968:
Prove that in every tetrahedron there is a vertex such that the three edges meeting there have lengths which are the sides of a triangle.
Threes and Fours
Misc
- The negative space in the eight of diamonds forms an 8.
- William Brewster, leader of the Plymouth Colony, named his children Jonathan, Patience, Fear, Love, and Wrestling.
- Wilfred Owen’s mother received the news of his death on Armistice Day.
- SCHOOLMASTER = SMOTE SCHOLAR
- “I never had any philosophic instruction, the first lecture on psychology I ever heard being the first I ever gave.” — William James
Good Boy
In 1919, engineer James Cowan Smith bequeathed £55,000 to the National Gallery of Scotland.
He set two conditions. One was that the gallery provide for his dog Fury.
The other was that this picture of his previous dog Callum, by painter John Emms, always be hung in the gallery.
Both conditions were fulfilled.
Seduction
In 1940 Bertrand Russell was invited to teach logic at the City College of New York.
A Mrs. Kay of Brooklyn opposed the appointment, citing Russell’s agnosticism and his alleged practice of sexual immorality.
In the lawsuit his works were described as “lecherous, libidinous, lustful, venerous, erotomaniac, aphrodisiac, irreverent, narrowminded, untruthful, and bereft of moral fiber.”
“Although he lost the case, the aging Russell was delighted to have been described as ‘aphrodisiac,'” writes Betsy Devine in Absolute Zero Gravity. “‘I cannot think of any predecessors,’ he claimed, ‘except Apuleius and Othello.'”
Dance Lessons
The quicksort computer sorting algorithm demonstrated with Hungarian folk dance, from Romania’s Sapientia University.
Also:
The four queens puzzle solved using ballet.
Binary search through flamenco dance.
Merge sort via Transylvanian-Saxon folk dance.
Selection sort using Gypsy folk dance.
(Via MetaFilter.)
01/19/2019 UPDATE: When Gavin Taylor showed these algorithms to his students at the United States Naval Academy, they asked whether they themselves could dance for extra credit. He said yes. So here are the U.S. Naval Academy midshipmen dancing the InsertionSort algorithm:
(Thanks, Gavin.)
Morrie’s Law
Richard Feynman was so struck by this fact that he remembered ever afterward where he had learned it — from his childhood friend Morrie Jacobs as the two stood in Morrie’s father’s leather shop in Far Rockaway, Queens.
Tempting Fate
What remained of the Tenth [Massachusetts] departed from City Point, on the James River, on June 21 [1864], for the return to Springfield and Northampton. But before leaving Virginia, on June 20, Sgt. Maj. George F. Polley, who was originally in Brewster’s company and had just reenlisted, carved his name and the inscription ‘Killed June –, 1864’ on a piece of board torn from a cracker box. After participating in the ‘goodbye’ rituals with his comrades and sharing an awkward amusement with them about his carving, Polley was struck flush by an artillery shell and killed. In his diary, brigade member Elisha Hunt Rhodes recorded this incident in his matter-of-fact style. Polley ‘showed me a board on which he had carved his name, date of birth and had left a place for the date of his death,’ reported Rhodes. ‘I asked him if he expected to be killed and he said no, and that he had made his head board only for fun. To day he was killed by a shell from a Rebel Battery.’ The last act of the Tenth before boarding the mailboat for Washington, D.C., was to bury Polley.
— David W. Blight, When This Cruel War Is Over, 2009
Area Matters
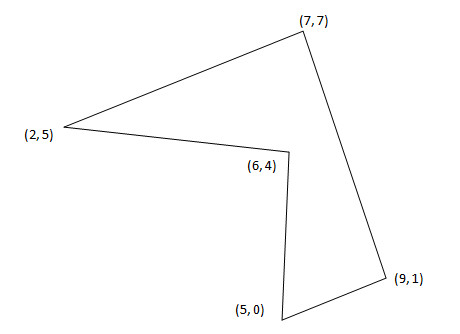
If you know the vertices of a polygon, here’s an interesting way to find its area:
- Arrange the vertices in a vertical list, repeating the first vertex at the end (see below).
- Multiply diagonally downward both ways as shown.
- Add the products on each side.
- Find the difference of these sums.
- Halve that difference to get the area.
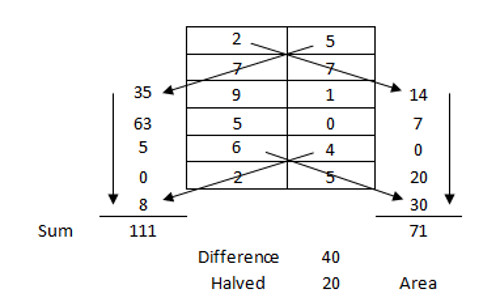
This works for any polygon, no matter the number of points, so long as it doesn’t intersect itself. It’s a slight restatement of the shoelace formula.
(Thanks, Derek, Dan, and Kyle.)
The Vowel Triangle
Chris McManus discovered this oddity. If W and Y are accepted as vowels, that gives us AEIOUWY. Starting with O, number these according to their positions on a circular alphabet without starting the count over for A (that is, O is the 15th letter of the alphabet, so it’s assigned number 15; beyond Z we’d reach A as the “27th” letter; and so on). Now write these numbers into a triangle, again starting with O:
O 15 U W Y 21 23 25 A E I 27 31 35
Each of the five lines in the figure gives a different arithmetic progression:
UWY: difference of 2
AEI: difference of 4
OUA: difference of 6
OWE: difference of 8
OYI: difference of 10
(David Morice, “Kickshaws,” Word Ways 34:4 [November 2001], 292-305.)
Podcast Episode 232: The Indomitable Spirit of Douglas Bader
Douglas Bader was beginning a promising career as a British fighter pilot when he lost both legs in a crash. But that didn’t stop him — he learned to use artificial legs and went on to become a top flying ace in World War II. In this week’s episode of the Futility Closet podcast we’ll review Bader’s inspiring story and the personal philosophy underlay it.
We’ll also revisit the year 536 and puzzle over the fate of a suitcase.