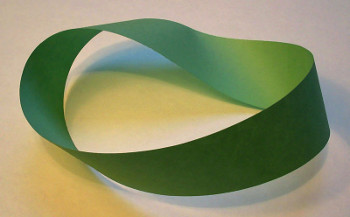
The properties of the simple Möbius strip are well understood: Take a strip of paper, give it a half-twist, and tape the ends together. Now an ant can traverse the full length of the loop, on both sides, and return to its starting point without ever crossing an edge.

But try doing the same thing with two strips of paper. Pair the strips, give them a half-twist, and connect the ends. Now it’s possible to insert a toothpick between the bands and to draw the toothpick along the entire length of the loop, which seems to show that they’re two distinct objects. But if you draw a line along either strip, starting anywhere, you’ll find that you traverse both strips and return to your starting point.

“I have known people to ponder this for hours while listening to Pink Floyd without ever fully appreciating what they have beheld,” writes Clifford Pickover in The Möbius Strip. Are you holding one object or two?